博文
一个开创性的数学方程式可能在未来会改变什么?
 精选
精选
||
一个开创性的数学方程式可能在未来会改变什么?
诸平
据英国布里斯托尔大学(University of Bristol)2022年9月6日提供的消息,开创性的数学公式为在健康、能源和食品工业方面取得令人兴奋的进步铺平了道路(Pioneering mathematical formula paves way for exciting advances in health, energy, and food industry)。一个具有开创性的数学方程被发现,它可能会改变未来的医疗程序、天然气开采和塑料包装生产。
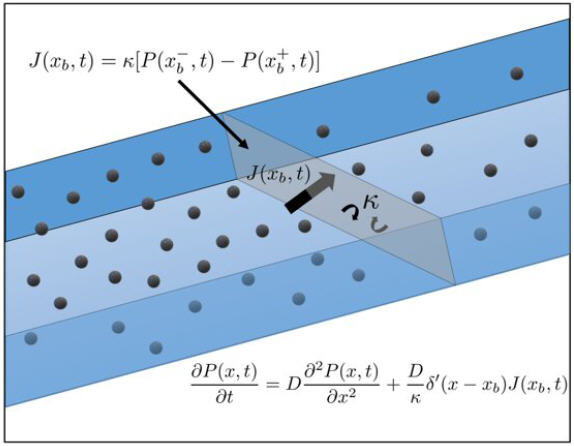
布里斯托尔大学科学家开发的新方程表明,通过渗透性材料的扩散运动首次可以精确建模。一个世纪前,世界顶尖物理学家阿尔伯特·爱因斯坦(Albert Einstein)和玛丽安·冯·斯莫卢乔夫斯基(Marian von Smoluchowski)推导出了第一个扩散方程,这标志着从微观粒子、自然生物到人造设备等各种实体在表示运动方面取得了重要进展。
到目前为止,科学家们在研究粒子通过多孔材料(如生物组织、聚合物、各种岩石和海绵)的运动时,不得不依赖近似或不完整的视角。
2022年9月7日发表在《物理评论研究》(Physical Review Research)杂志网站的研究结果提供了一种新的技术,为健康、能源和食品行业等各种环境提供了令人兴奋的机会。详见Toby Kay, Luca Giuggioli. Diffusion through permeable interfaces: Fundamental equations and their application to first-passage and local time statistics. Physical Review Research, 2022, 4: L032039 – Published 7 September 2022. https://doi.org/10.1103/PhysRevResearch.4.L032039
正在攻读工程数学博士学位托比·凯(Toby Kay)是这篇论文的主要作者。他说:“这标志着自阿尔伯特·爱因斯坦和玛丽安·冯·斯莫卢乔夫斯基对扩散的研究以来,迈出了重要的一步。它彻底改变了通过各种规模的复杂介质(从细胞成分和地质化合物到环境栖息地)对扩散实体的建模。
在此之前,用数学方法来描述在分散有阻碍运动物体的环境中的运动,也就是所谓的可渗透屏障(permeable barriers),都是有限的。通过解决这个问题,我们正在为许多不同领域令人兴奋的进步铺平道路,因为可渗透屏障是动物、细胞生物和人类经常遇到的。”
数学中的创造力有不同的表现形式,其中之一是对现象不同层次的描述之间的联系。在这种情况下,通过以微观方式表示随机运动,然后缩小到宏观描述过程,就有可能找到新的方程式。
需要进一步研究,将这种数学工具应用到实验应用中,以改进产品和服务。例如,能够精确地模拟水分子通过生物组织的扩散,将促进扩散加权磁共振成像(Magnetic Resonance Imaging简称MRI)读数的解释。它还可以更准确地描述空气通过食品包装材料传播的情况,帮助确定保质期和污染风险。此外,量化觅食动物与宏观屏障(如栅栏和道路)相互作用的行为,可以更好地预测气候变化的后果,以达到保护目的。
地理定位器(geolocators)、移动电话和其他传感器的使用见证了跟踪革命在过去20年中产生的移动数据的数量和质量不断增加。这突出表明需要更复杂的建模工具来表示环境中各种实体的运动,从自然生物到人造设备。
通讯作者、布里斯托尔大学复杂性科学副教授卢卡·朱吉奥利博士(Dr. Luca Giuggioli)说:“这一新的基本方程是另一个例子,说明了在空间异质性的情况下,即当底层环境从一个位置到另一个位置发生变化时,构建工具和技术来表示扩散的重要性。
它建立在另一个期待已久的解决方案的基础上,该解决方案将于2020年解决一个数学难题,以描述受限空间中的随机运动。这一最新发现是进一步提高我们对所有形状和形式的运动的理解的重要一步,统称为运动数学(mathematics of movement),它有许多令人兴奋的潜在应用。”
上述介绍,仅供参考。欲了解更多信息,敬请注意浏览原文或者相关报道。
解决百年数学难题,可预测传染病的传播(Solution to century-old math problem could predict transmission of infectious diseases)
The diffusion equation is the primary tool to study the movement dynamics of a free Brownian particle, but when spatial heterogeneities in the form of permeable interfaces are present, no fundamental equation has been derived. Here we obtain such an equation from a microscopic description using a lattice random walk model. The sought after Fokker-Planck description and the corresponding backward Kolmogorov equation are employed to investigate first-passage and local time statistics and gain new insights. Among them a surprising phenomenon, in the case of a semibounded domain, is the appearance of a regime of dependence and independence on the location of the permeable barrier in the mean first-passage time. The new formalism is completely general: it allows to study the dynamics in the presence of multiple permeable barriers as well as reactive heterogeneities in bounded or unbounded domains and under the influence of external forces.
https://blog.sciencenet.cn/blog-212210-1354778.html
上一篇:科学家们在二维磁体中看到自旋
下一篇:没有奶牛的真牛奶的未来