博文
文章荐读 | 基于比例犹豫模糊语言优先Choquet聚合算子的教学成果评价
||
小编导读
多专家教学成果评价是一个多属性群决策问题,可以提高教学质量。来自山东财经大学和山东电视大学的学者们在期刊 International Journal of Computational Intelligence Systems(IJCIS) 上发表了题为“Teaching Performance Evaluation Based on the Proportional Hesitant Fuzzy Linguistic Prioritized Choquet Aggregation Operator”的文章,研究了基于比例犹豫模糊语言优先Choquet聚合算子的教学成果评价。
要点介绍
近年来,中外合作办学项目是我国跨国教育的主要形式。随着学校规模的不断扩大和项目运行的逐步升级,学校的可持续发展已成为必然要求,这有赖于教学质量的提高。教师素质、教学方法、教学能力已成为影响教学质量的因素。教学成果评价可以由一组基于多属性的决策者来进行,这些决策者可能具有不精确性、模糊性和不确定性。因此,中外合作办学项目的教学成果评价可以看作是一个模糊多属性群决策问题。全面有效地运用科学的方法对教学成果评价的质量起着至关重要的作用。
本文提出了一种在比例犹豫模糊语言环境下进行教学成果评价的群体决策方法。首先,利用比例犹豫模糊语言术语集(PHFLTS)表达决策者对教学成果指标的偏好。其次,发展了PHFLPr-CA算子并讨论了其性质。然后基于PHFLPrCA算子,提出了一种MAGDM方法。再次,将该方法应用于中外合作办学项目的教学成果评价中。通过与其他两种方法的比较,证明了该方法的科学性、客观性和准确性。
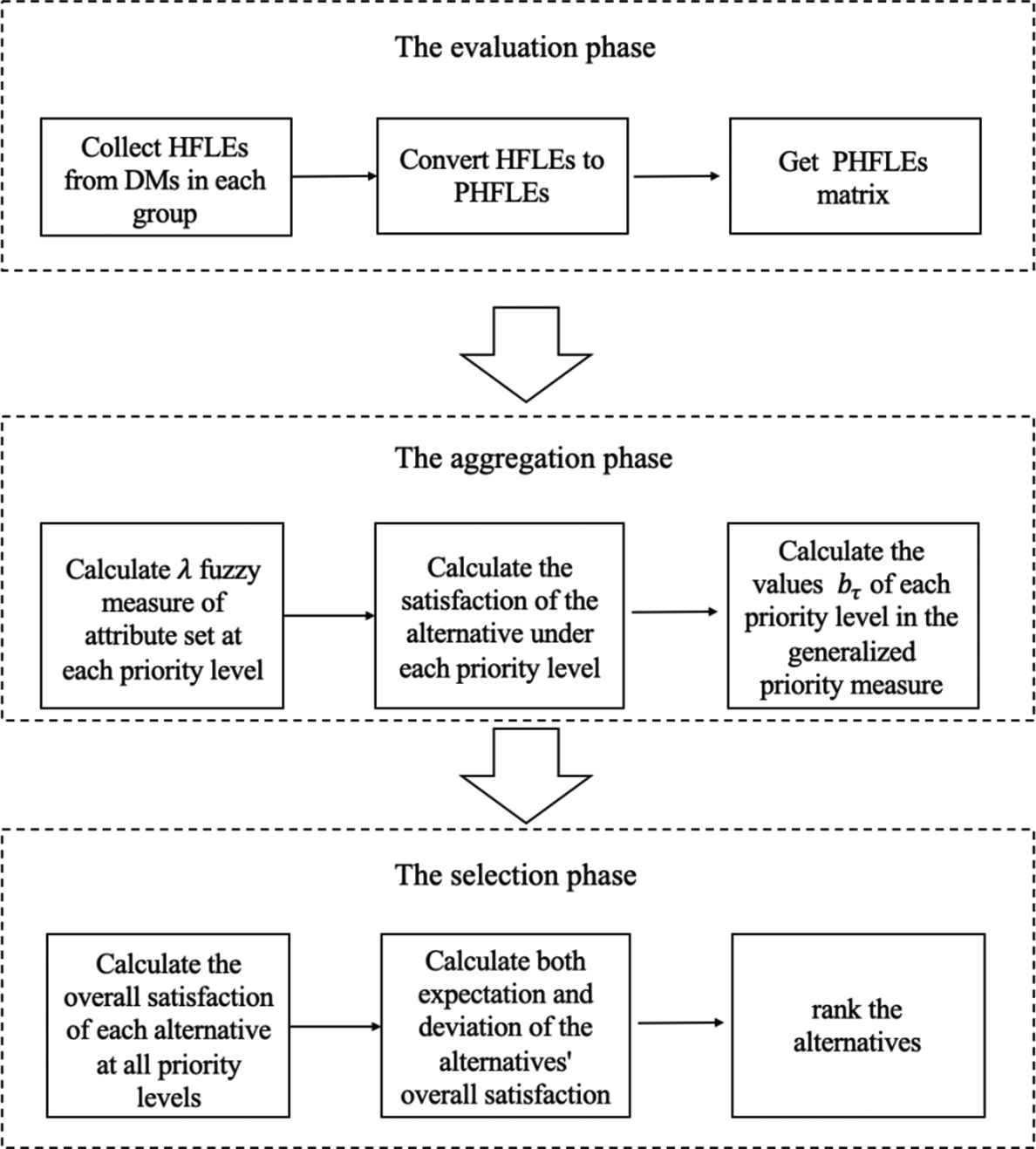
图1. 基于PHFLPrCA算子的多属性群决策方法框架
研究结论:为了解决中外合作办学项目教学成果评价问题,本文主要研究了具有优先权和相关函数的PHFLE信息聚合算子及其应用。首先,针对属性间存在相关性(冗余、互补和独立)的MAGDM问题,提出了PHFLCAg算子。其次,针对属性同时具有优先级和相关性的情况,提出了PHFLPrCA算子。本文简要介绍了相关算子的性质,并提出了相应的算子。第三,基于PHFLPrCA算子,提出了一种新的MAGDM方法。为了验证该方法的有效性,本文选取了S学院中外合作办学项目教学成果评价中具有优先性和相关性的典型MAGDM问题,并且阐述了该方法在解决问题中的作用。最后,通过与其他两种方法的比较,说明了该方法的优越性。
局限性:第一个限制是在平衡和对称的环境中假设的LTS,然而在现实生活中,LTS有时是不平衡和不对称的。第二个限制是决策者采用相同的策略,但决策者往往根据自己的经验和偏好选择不同的策略。未来PHFLE将应用于非平衡多颗粒环境。
参考文献 References
[1] J.Chen,H.Hsieh,Q.Do, Evaluating teaching performance based on fuzzy AHP and comprehensive evaluation approach, Appl. Soft Comput. 28 (2015), 100–108.
[2] Z.S. Chen, M. Li, W.T. Kong, K.S. Chin, Evaluation and selection of hazmat transportation alternatives: a PHFLTS- and TOPSIS- integrated multi-perspective approach, Int. J. Environ. Res. Pub. Health. 16 (2019), 4116.
[3] L.Zhou,H.Li,K.Sun,Teaching performance evaluation by means of a hierarchical multifactorial evaluation model based on type-2 fuzzy sets, Appl. Intell. 46 (2016), 34–44.
[4] M.Kuzmanovic,G.Savic,B.AndricGusavac,D.Makajic-Nikolic, B. Panic, A conjoint-based approach to student evaluations of teaching performance, Expert Syst. Appl. 40 (2013), 4083–4089.
[5] B. Dutta, Á. Labella, R.M. Rodríguez, L. Martínez, Aggregat- ing interrelated attributes in multi-attribute decision-making with ELICIT information based on Bonferroni mean and its variants, Int. J. Comput. Intell. Syst. 12 (2019), 1179–1196.
[6] Á. Labella, R.M. Rodríguez, L. Martínez, Computing with com- parative linguistic expressions and symbolic translation for deci- sion making: ELICIT information, IEEE Trans. Fuzzy Syst. 28 (2020), 2510–2522.
[7] S.H. Xiong, Z.S. Chen, K.S. Chin, A novel MAGDM approach with proportional hesitant fuzzy sets, Int. J. Comput. Intell. Syst. 11 (2018), 256–271.
[8] P. Liu, Y. Wang, Intuitionistic fuzzy interaction hamy mean operators and their application to multi-attribute group decision making, Group Decis. Negot. 28 (2019), 197–232.
[9] P.Liu,W.Liu,ScaledPrioritizedOperatorsBasedontheLinguistic Intuitionistic Fuzzy Numbers and Their Applications to Multi- Attribute Decision Making, Int. J. Fuzzy Syst. 20 (2018), 1539– 1550.
[10] X.Liang,P.Liu,Z.Wang,Hotelselectionutilizingonlinereviews: a novel decision support model based on sentiment analysis and dl-vikor method, Technol. Econ. Dev. Econ. 25 (2019), 1139–1161.
[11] X. Liang, F. Teng, Y. Sun, Multiple group decision making for selecting emergency alternatives: a novel method based on the ldwpa operator and ld-mabac, Int. J. Environ. Res. Pub. Health. 17 (2020), 2945.
[12] Z.S.Chen,K.S.Chin,Y.L.Li,Y.Yang,Proportionalhesitantfuzzy linguistic term set for multiple criteria group decision making, Inf. Sci. 357 (2016), 61–87.
[13] Z.S.Chen,Y.Yang,X.J.Wang,K.S.Chin,K.L.Tsui,Fosteringlin- guistic decision-making under uncertainty: a proportional inter- val type-2 hesitant fuzzy TOPSIS approach based on Hamacher aggregation operators and andness optimization models, Inf. Sci. 500 (2019), 229–258.
[14] R.M. Rodríguez, Á. Labella, G. De Tré, L. Martínez, A large scale consensus reaching process managing group hesitation, Knowl. Based Syst. 159 (2018), 86–97.
[15] J. Huang, X.Y. You, H.C. Liu, S.L. Si, New approach for quality function deployment based on proportional hesitant fuzzy lin- guistic term sets and prospect theory, Int. J. Prod. Res. 57 (2019), 1283–1299.
[16] L.A.Zadeh, The concept of a linguistic variable and its application to approximate reasoning-I, Inf. Sci. 8 (1975), 199–249.
[17] L.A.Zadeh,Theconceptofalinguisticvariableanditsapplication to approximate reasoning-II, Inf. Sci. 8 (1975), 301–357.
[18] L.A.Zadeh,Theconceptofalinguisticvariableanditsapplication to approximate reasoning-III, Inf. Sci. 9 (1975), 43–80.
[19] R.M. Rodriguez, L. Martinez, F. Herrera, A group decision making model dealing with comparative linguistic expressions based on hesitant fuzzy linguistic term sets, Inf. Sci. 241 (2013), 28–42.
[20] H. Liao, Z. Xu, X.J. Zeng, Hesitant fuzzy linguistic vikor method and its application in qualitative multiple criteria decision mak- ing, Fuzzy Syst. IEEE Trans. 23 (2015), 1343–1355.
[21] H. Wang, Z. Xu, Total orders of extended hesitant fuzzy linguistic term sets: definitions, generations and applications, Knowl. Based Syst. 107 (2016), 142–154.
[22] C. Wei, R.M. Rodriguez, L. Martinez, Uncertainty measures of extended hesitant fuzzy linguistic term sets, IEEE Trans. Fuzzy Syst. 26 (2018), 1763–1768.
[23] S.M. Yu, J. Wang, J.Q. Wang, L. Li, A multi-criteria decision- making model for hotel selection with linguistic distribution assessments, Appl. Soft Comput. 67 (2017), 741–755.
[24] G. Zhang, Y. Dong, Y. Xu, Consistency and consensus measures for linguistic preference relations based on distribution assess- ments, Inf. Fusion. 17 (2014), 46–55.
[25] M. Grabisch, Fuzzy integral in multicriteria decision making, Fuzzy Sets Syst. 69 (1995), 279–298.
[26] R.R. Yager, Prioritized aggregation operators, Int. J. Approx. Rea- son. 48 (2008), 263–274.
[27] Z.S. Chen, X.L. Liu, R.M. Rodríguez, X.J. Wang, K.S. Chin, K.L. Tsui, L. Martínez, Identifying and prioritizing factors affect- ing in-cabin passenger comfort on high-speed rail in China: a fuzzy-based linguistic approach, Appl. Soft Comput. 95 (2020), 106558.
[28] P. Liu, Y. Wang, Interval neutrosophic prioritized owa operator and its application to multiple attribute decision making, J. Syst. Sci. Compl. 29 (2016), 681–697.
[29] P. Liu, Y. Li, J. AntucheviČiene, Multi-criteria decision-making method based on intuitionistic trapezoidal fuzzy prioritised owa operator, Technol. Econ. Dev. Econ. 22 (2016), 453–469.
[30] P. Liu, J. Liu, Some q-rung orthopai fuzzy bonferroni mean oper- ators and their application to multi-attribute group decision mak- ing, Int. J. Intell. Syst. 33 (2018), 2341–2363.
[31] M. Sugeno, Fuzzy measure and fuzzy integral, Trans. Soc. Instr. Control Eng. 8 (1972), 218–226.
[32] F. Herrera, E. Herrera-Viedma, J.L. Verdegay, A model of consen- sus in group decision making under linguistic assessments, Fuzzy Sets Syst. 78 (1996), 73–87.
[33] R.M. Rodriguez, L. Martinez, F. Herrera, Hesitant fuzzy linguistic term sets for decision making, IEEE Trans. Fuzzy Syst. 20 (2012), 109–119.
[34] Q. Pang, H. Wang, Z. Xu, Probabilistic linguistic term sets in multi-attribute group decision making, Information Sciences, 369 (2016), 128–143.
[35] M. Grabisch, I. Kojadinovic, P. Meyer, A review of methods for capacity identification in Choquet integral based multi-attribute utility theory: applications of the Kappalab R package, Eur. J. Oper. Res. 186 (2008), 766–785.
[36] M. Grabisch, The application of fuzzy integrals in multicriteria decision making, Eur. J. Oper. Res. 89 (1996), 445–456.
[37] L. Chen, Z. Xu, X. Yu, Prioritized measure-guided aggregation operators, IEEE Trans. Fuzzy Syst. 22 (2014), 1127–1138.[38] X. Wang, E. Triantaphyllou, Ranking irregularities when evaluat- ing alternatives by using some electre methods, Omega. 36 (2008), 45–63.
原文信息
L. Wang, L. Rong, F. Teng, P. Liu, "Teaching Performance Evaluation Based on the Proportional Hesitant Fuzzy Linguistic Prioritized Choquet Aggregation Operator", International Journal of Computational Intelligence Systems, 2020, DOI: https://doi.org/10.2991/ijcis.d.210112.001.

扫描二维码,获取英文原文
https://www.atlantis-press.com/journals/ijcis/125951265
关于期刊

Impact Factor: 1.838, CiteScore: 3.59
International Journal of Computational Intelligence Systems(IJCIS)是欧洲模糊逻辑和技术学(EUSFLAT)会刊,主要刊载有关应用计算智能各个方面的原创性研究,尤其是针对证明使用了计算智能理论的技术和方法的研究型论文及综述等,由西班牙哈恩大学Luis Martínez Lopez教授和澳大利亚悉尼科技大学路节教授担任共同主编。本刊目前已被DOAJ, Science Citation Index Expanded (SCIE), Ei Compendex and Scopus等数据库收录。

Atlantis Press是一家出版科学、技术和医学(STM)内容的全球开放获取出版机构,2006年成立于法国巴黎。现总部位于荷兰阿姆斯特丹,在法国巴黎及中国的北京、郑州和香港设有办事处。我们的使命是通过促进科研界和整个社会更有效地传播和交流知识来支持科学、技术和医学研究的进步。迄今,Atlantis Press的数字内容平台包含超过14万篇开放获取论文供读者免费下载阅读,每年产生数千万下载量。Atlantis Press是施普林格•自然集团的一部分。
https://blog.sciencenet.cn/blog-3453320-1280928.html
上一篇:Atlantis Press正式并入Springer Nature
下一篇:文章荐读 | 基于金属有机框架(MOFs)的食品安全评价分析技术