博文
清华笔记:计算共形几何讲义 (17)全纯二次微分(holomorphic quadratic differential)
 精选
精选
|

【上课时间:每周二和周四上午9:50-11:20AM;地点:清华大学,近春园西楼三楼报告厅。欢迎任何有兴趣的朋友,前来旁听指导。】
上次课程,我们讲解了调和映照的理论框架。这次课程,我们应用从度量曲面到带度量的图的调和映照来计算全纯二次微分。全纯二次微分和曲面上的叶状结构和曲面间拟共形映射具有紧密的联系。
全纯二次微分和叶状结构的理论历史发展如下:Hubbard-Masur (【7】1979)证明了全纯二次微分和叶状结构的等价性;Jenkin(【3】1957)和Strebel (【4】1984)证明了满足特定组合、几何条件的全纯二次微分的存在性;Wolf(【5】1996)证明了全纯二次微分可以由广义调和映照得到;Schone-Gromove (【6】1992)证明了广义调和映照的存在性和唯一性。
全纯一形式
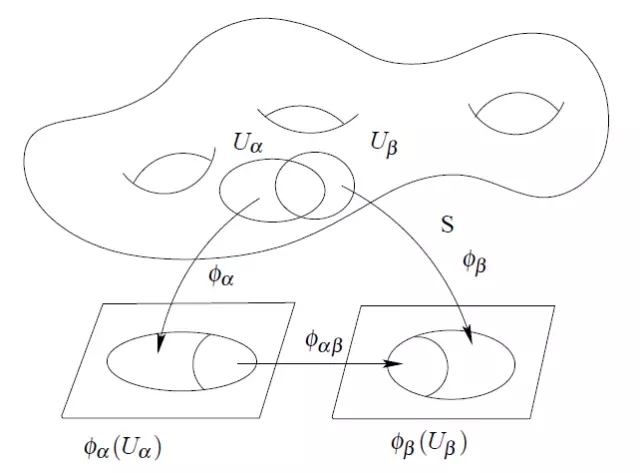
图1. 黎曼面。


图2. 亏格为二的曲面上全纯一形式的基底。

全纯二次微分

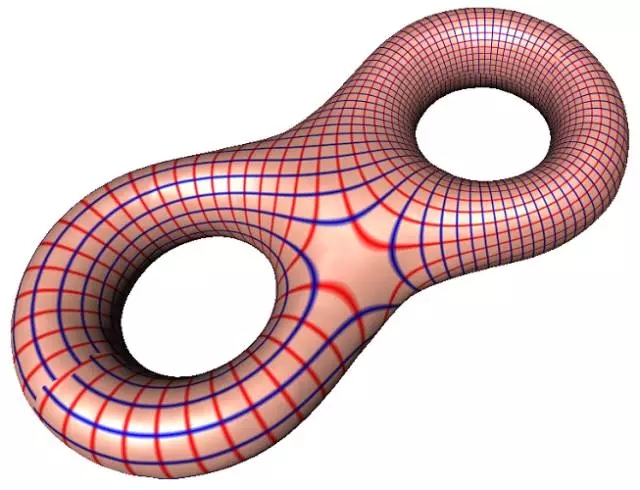
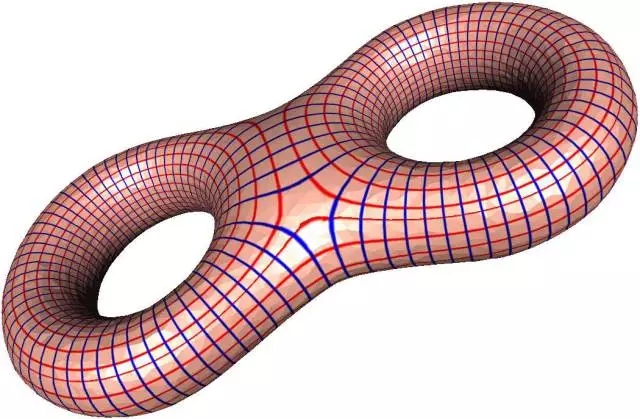
图3.全纯一形式的轨线和全纯二次微分的轨线对比。

叶状结构


图4. 亏格为3的曲面上的叶状结构。

广义调和映照


图5. 可容许曲线系统(Admissible Curve System)【2】。

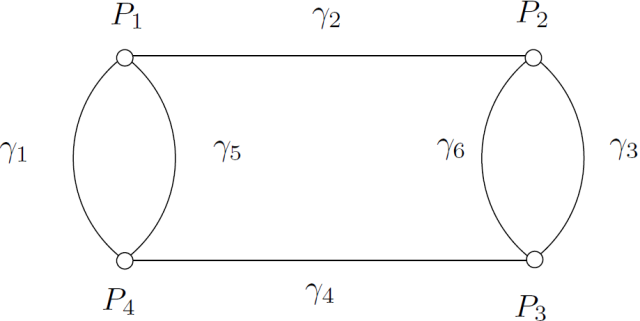
图6. 裤子分解图(Pants Decomposition Graph)。【2】


图7. 曲面上的叶状结构(foliation)。【2】


图8. Cylindric Decomposition。【2】
图9. 全纯二次微分。(holomorphic quadratic differential)【2】
References
1. N. Lei, X. Zheng, J. Jiang, Y.-Y. Lin and X. Gu, Quadrilateral and Hexahedral mesh generation based on surface foliation theory I, Computer Methods in Applied Mechanics and Engineering. 2017 (316), 758-781.
2. N. Lei, X. Zheng, Z. Luo and X. Gu, Quadrilateral and Hexahedral mesh generation based on surface foliation theory II, Computer Methods in Applied Mechanics and Engineering. In press, 2017.
3. J. A. Jenkins, On the existence of certain general extremal metrics, Ann.
595 of Math. 60 (1957) 440–453.
4. K. Strebel, Quadratic Differentials, Springer-Verlag, 1984.
5. M. Wolf, On realizing measured foliations via quadratic differentials of
harmonic maps to r-trees, J. D’Analyse Math (1996) 107–120.
6. M. Gromov, R. Schoen, Harmonic maps into singular spaces and p-adic
superrigidity for lattices in groups of rank one, Publ. Math. IHES 76 (1992) 165–246.
7. J.Hubbard,H.Masur,Quadrtic Differentials and foliations, Acta Math. (142) (1979) 221-274.
https://blog.sciencenet.cn/blog-2472277-1199143.html
上一篇:清华笔记:计算共形几何讲义 (16)拓扑球面的调和映照
下一篇:清华笔记:计算共形几何讲义 (18)拟共形映射(Quasi-Conformal Map)
全部作者的精选博文
全部作者的其他最新博文
- • 清华笔记:计算共形几何讲义 (23)离散曲面曲率流 (Discrete Surface Ricci Flow)V
- • 清华笔记:计算共形几何讲义 (22)离散曲面曲率流 (Discrete Surface Ricci Flow)IV
- • 清华笔记:计算共形几何讲义 (21)离散曲面曲率流 (Discrete Surface Ricci Flow)III
- • 清华笔记:计算共形几何讲义 (20)离散曲面曲率流 (Discrete Surface Ricci Flow)II
- • 清华笔记:计算共形几何讲义 (19)离散曲面曲率流 (Discrete Surface Ricci Flow ) I
- • 清华笔记:计算共形几何讲义 (18)拟共形映射(Quasi-Conformal Map)