博文
《物理世界》研究进展: 可抑制量子计算误差的新型准粒子获实验演示
|

EXPRESS LETTER
Digital Simulation of Projective Non-Abelian Anyons with 68 Superconducting Qubits
Shibo Xu, Zheng-Zhi Sun, Ke Wang, Liang Xiang, Zehang Bao, Zitian Zhu, Fanhao Shen, Zixuan Song, Pengfei Zhang, Wenhui Ren, Xu Zhang, Hang Dong, Jinfeng Deng, Jiachen Chen, Yaozu Wu, Ziqi Tan, Yu Gao, Feitong Jin, Xuhao Zhu, Chuanyu Zhang, Ning Wang, Yiren Zou, Jiarun Zhong, Aosai Zhang, Weikang Li, Wenjie Jiang, Li-Wei Yu, Yunyan Yao, Zhen Wang, Hekang Li, Qiujiang Guo, Chao Song, H. Wang, and Dong-Ling Deng
Chin. Phys. Lett. 2023, 40 (6): 060301
DOI: 10.1088/0256-307X/40/6/060301
研究快讯
超导系统中实现非阿贝尔任意子的量子模拟
浙江大学物理学院宋超、王浩华研究组与清华大学交叉信息研究院邓东灵研究组等合作,在超导系统中实验实现了非阿贝尔任意子的数字化量子模拟。
Philip Ball in Physics World wrote a research update that highlights this work, and the other two .
https://physicsworld.com/a/new-type-of-quasiparticle-emerges-to-tame-quantum-computing-errors/
New type of quasiparticle emerges to tame quantum computing errors

Topological manipulations: A graphic representing non-Abelian braiding of graph vertices in a superconducting quantum processor. (Courtesy: Google Quantum AI)
Errors are the Achilles’ heel of quantum computation, cropping up at random and threatening to ruin calculations. But they might, in principle, be tamed by encoding quantum information in a type of quasiparticle called a non-Abelian anyon. Evidence that such quasiparticles may exist has now been reported independently by teams at Google, Microsoft, the quantum-computing firm Quantinuum, and Zhejiang University in China.
The new reports “make a very intriguing advance in quantum computing”, says Jiannis Pachos, a physicist the University of Leeds, UK. “They are all things we have been waiting a long time to see,” agrees Steven Simon, a theorist at the University of Oxford, UK. “It’s a very exciting time for the field.” Still, Simon cautions that none of the results will transform quantum computing yet. “They all have shortcomings, which means there is lots of room for further work,” he says.
Errors in quantum computing
Quantum computers encode binary information in quantum bits, or qubits, which can take on values of 1, 0 or a superposition of the two. Various physical systems can act as qubits, including ions, photons and tiny components made from superconducting material. Quantum computations are performed by entangling many qubits so that their states are interdependent, then manipulating them according to some algorithm before reading out their final states.
The trouble is that random noise in the environment can change qubit states during the computation, making the calculation less reliable. This can happen in classical computers too, but there the problem is relatively easy to solve, for example by keeping several copies of each bit and assigning its value by majority rule. That won’t work for qubits, however, because quantum mechanics prohibits the copying of unknown quantum states, while the very nature of quantum computing requires that the qubit states remain unknown during the computation. As a result, researchers have had to search for more complicated methods of error correction.
Majorana particles to the rescue
An alternative approach is to use qubits that are more resistant to errors. In a paper written in 1997 (and published in 2003), physicist Alexei Kitaev showed that it might be possible to create error-protected qubits from hypothetical entities called Majorana particles. First proposed in 1937 by the physicist Ettore Majorana, these particles have a peculiar property: they are their own antiparticle.
No one knows whether Majorana particles exist as fundamental particles. However, Kitaev showed that they can, in principle, be created from collective states of electrons called quasiparticles. He also showed that, if used as qubits, these states would be “topologically protected”, meaning that they can’t be randomly flipped by noise without “breaking” the quasiparticle, just as you can’t get rid of the twist in a Möbius strip without cutting it. Kitaev later proposed that these Majorana quasiparticles might be engineered as electronic defect states at the ends of quantum nanowires made from (for example) a semiconductor situated close to a superconductor.

Hunting for MZMs: Azure Quantum Hardware engineer Ajuan Cui works on equipment in Microsoft’s Quantum Materials Lab (Courtesy: John Brecher/Microsoft)
These defect states are known as Majorana zero modes (MZMs), and they belong to a class of hypothetical (quasi)particles termed non-Abelian anyons. The “anyon” part signifies that the particles are neither fermions nor bosons – a property hypothesized by physicist Frank Wilzcek in 1982 and eventually observed (by teams in the US and France) in 2020 in electronic quasiparticles. Those observations, however, were of Abelian anyons, which are different from the non-Abelian variety Kitaev proposed for error-protected qubits. Specifically, if two non-Abelian anyons exchange places, their quantum states change in a detectable way even though the particles themselves are identical. Abelian anyons, in contrast, acquire no such observable change – only their quantum phase is altered.
The tangled web we weave
To make qubits from non-Abelian anyons, Kitaev suggested moving the anyons around to weave their trajectories together. This weaving process is called braiding, and it lets the anyons swap places in a way that alters their observable states. This can then be used to perform a logic operation. Enacting a quantum algorithm thus entails braiding non-Abelian anyons in a particular way, then reading out the result.
The main benefit of this set-up is that, in effect, the topology of the braiding “remembers” the qubit states. Errors can only arise if a braid is cut, which requires a lot of energy. Under these circumstances, the computation is said to be topologically error-corrected.
In 2018, researchers in China and the UK (including Pachos) simulated the braiding of non-Abelian MZMs in an optical system. In that experiment, the quantum states corresponded to photon polarization states. The most recent results take this a step further by showing how to implement the idea in real many-qubit quantum circuits.
The difficult task of making MZMs
While many groups have pressed ahead with making quantum computers from conventional, non-Majorana qubits supported by quantum error-correcting codes, Microsoft has pinned its quantum computing hopes on making topologically protected qubits from MZMs. “Microsoft’s longstanding belief is that engineering an error-protected topological qubit is the path to delivering quantum computing at scale”, says Chetan Nayak of Microsoft Quantum in Santa Barbara, California. “In engineering a topological qubit directly in the hardware, we will be able to create a new type of qubit that is fast, small, and controllable.”
Hardware guy: Chetan Nayak leads the hardware programme at Microsoft’s Azure Quantum. (Courtesy: John Brecher/Microsoft)
But this has proved extremely hard, and the field has been dogged with claims that have subsequently crumbled under scrutiny. Part of the challenge has been to identify a clear signature of MZMs that distinguishes them from other quasiparticles. In their latest paper, Nayak and colleagues report evidence for what they think is a discriminating criterion for MZMs. This criterion is known as the topological gap protocol, and the Microsoft researchers say that their system – a thin film of semiconducting indium arsenide sitting below a 120-nanometre-wide strip of superconducting aluminium – passes it.
Making MZMs in such tiny objects would come with a big advantage. “In principle you could put a hell of a lot of them on a chip,” says Simon. However, the Microsoft paper is not yet peer-reviewed, and the claim will need to be checked carefully. “The hunt for MZMs has over-promised many times,” Simon adds. Though the new result looks promising, he suspects that, ultimately, claims for MZMs will only be accepted “if you can make and manipulate a qubit” from them. “Show me a qubit and then we’ll talk,” he says.
Anyons or simulations of anyons?
Meanwhile, teams at Google Quantum AI in the US, Zhejiang University in Hangzhou, China, and Quantinuum (formerly Honeywell Quantum Solutions and Cambridge Quantum Computing) in Germany and the US all claim to have made non-Abelian anyons from clever combinations of more conventional qubits – superconducting circuits for the Google and Zhejiang groups, and trapped ions for Quantinuum.
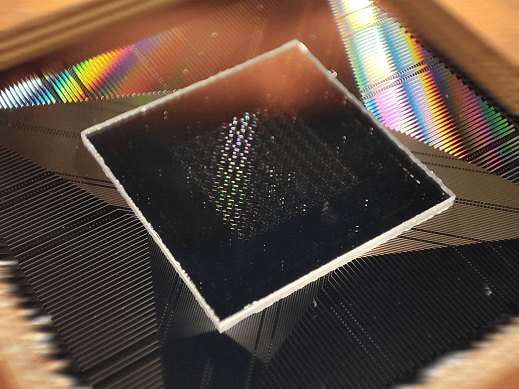
Superconducting array: The 68-qubit array used in the Zhejiang University team’s experiment. (Courtesy: Song Chao)
The question here is the meaning of the word “made”. In some sense, one could say that all three results involve not anyons as such but quantum simulations of them – a bit like simulating atoms on a classical computer. But the distinction is blurry. Because qubits are themselves quantum objects, they can be used to build exactly the same quantum wavefunction that an anyon would have. “It’s a fuzzy distinction between simulating matter and having matter,” says Simon. “But what they can say for sure is that they’ve made the wavefunction they want.”

Cool devices: Google Quantum AI hardware engineer Catherine Erickson works on the dilution refrigerator used to cool the quantum-computing chip to the temperature necessary to distinguish the quantized energy levels of the qubits. (Courtesy: Rocco Ceselin)
The Google and Zhejiang teams used similar approaches on, respectively, a 25-qubit chip and a 68-qubit array. In both experiments, the anyons correspond to defects in a square lattice of interacting qubits, a little like dislocations in a crystal lattice. The lowest-energy (ground) state of this lattice structure consists of wavefunctions corresponding to Abelian anyons – and as Kitaev explained, this in itself can be used to construct an error-correcting qubit protected with an error-correcting code called the surface code.
To make defects corresponding to error-protected non-Abelian anyons, the researchers manipulated the interactions between neighbouring qubits in a controlled sequence. Both teams show that the resulting quasiparticles can be braided by moving them around one another. The Google researchers, led by Pedram Roushan and Trond I Andersen, also created a well-known quantum-entangled state (a Greenberger-Horne-Zeilinger or GHZ state) from their anyons – a proof of principle for how the quasiparticles can be manipulated.
Another route to non-Abelian anyons
The Quantinuum group, meanwhile, made non-Abelian anyons in a different way. Using the Honeywell 32-qubit H2 quantum processor, which holds ytterbium ions in an electromagnetic trap and alters their quantum states using lasers, they created a quasi-one-dimensional chain of interacting trapped-ion qubits.
Here, the anyons correspond to natural excitations of the ground state of the qubit system – which technically means they are not quasiparticles, since quasiparticles must be excited states. “The Majorana zero modes at the end of superconducting wires in the Microsoft experiment and the lattice defects in the Google experiment are non-Abelian defects,” emphasizes Ashvin Vishwanath of Harvard University, who collaborated with the Quantinuum team. “Unlike our experiment, they are not realized on top of true non-Abelian topological order.”

Trapped ions: The Quantinuum H2 processor. (Courtesy: Quantinuum)
Nevertheless, all three results are “neat quantum simulations”, says Chris Monroe, a physicist at Duke University in North Carolina, US and chief scientist of the ion-trap quantum-computing company IonQ. He adds that such experiments could probably have been done on quantum-computing platforms years ago, but they have likely been motivated by recent interest in (and controversy about) MZMs in solid-state systems. “It’s a pretty interesting confluence of science and sociology,” he says.
Not yet universal
In Roushan’s view, the two approaches to braiding non-Abelian anyons – using superconducting qubits and trapped ions – are complementary. “There are advantages and disadvantages to each approach, but it is too early to say exactly how they will impact the respective developments,” he says.
In any event, both approaches are perhaps best regarded as proofs that non-Abelian anyon states – that is, the corresponding wavefunctions – exist. But Pachos warns that this is just a first step. “It is not clear yet how this can be moved to fault tolerance,” he says.
What is more, because these anyon states are made from conventional, error-prone qubits, no-one yet knows whether they can be made stable enough to truly act as topologically protected qubits. “To achieve fault tolerance, active error-correcting techniques will be required for our quantum simulation method,” acknowledges Song Chao, a physicist in the Zhejiang team. “A true breakthrough on the technology side will require showing that these protected qubits are more robust than the underlying qubits they are built from,” Vishwanath adds.
A further caveat is that none of the current experiments makes anyons with the right properties to provide qubits for “universal” quantum computing, which can embody any quantum algorithm. “They do have computational abilities, but none are universal,” Simon explains.
Still, all of these approaches “give a clear way to move forward”, says Pachos. He adds that “the field is very tense, as the conclusive discovery of [physical] non-Abelian anyons will lead to a Nobel prize”. But we are not there yet.
• This article was amended on 23/5/2023 to clarify Pedram Roushan’s comment on complementary approaches, and on 30/05/2023 to correct a reference to work done in 2018 by Jiannis Pachos and colleagues.
© Copyright 2023 IOP Publishing Ltd
研究快讯集锦
扭角双层GaTe中的光学可调控的摩尔激子
利用外加磁场调控材料的热膨胀系数
多组份玻色超流系统的流体力学
超导系统中实现非阿贝尔任意子的量子模拟
钽基富氢化物高压合成和高压超导的发现
利用解纠缠张量强化密度矩阵重整化群算法
基于第一性原理计算的Lu-H-N相图
基于范德华A型反铁磁CrSBr的自旋过滤隧道结中的巨大隧穿磁电阻
双层1T-TiSe2中的电子-激子耦合
Fe基Kagome晶格化合物单晶Y0.5Fe3Sn3的结构确定、非稳反铁磁态及输运行为
π介子质量分布的实验确定
700 Wh/kg锂二次软包电池
转角四层二硫化钼莫尔超晶格中铁电序与关联态的耦合
LuH2压力诱导的颜色变化
门电压可调的细直径InAs-Al纳米线超导量子比特
手性磁畴壁调控量子反常霍尔效应
“12442”型铁基超导体RbCa2Fe4As4F2中奇异的第二峰效应
利用时间合成维度构建光学神经网络
基于随机态含时演化的大尺度密度泛函理论计算方法
插层铁基(Li,Fe)OHFeSe:准二维高Tc超导电性
强场电离诱导的电子-空穴相干性实时测量
含记忆临界现象理论
光学克尔非线性谐振腔中的动态非互易
范德华铁磁/半导体异质结中的大室温磁电阻
准二维铁硒基超导体中普适赝能隙行为的核磁共振实验证据
兆巴高压下的金刚石NV中心光探磁共振
在超导量子电路中演示非阿贝尔系统的量子几何张量测量
基于里德堡原子基态封锁的双量子比特几何门
SrTiO3单晶立方相到四方相转变引起的弹性异常
氮化二聚钴:一类潜在的新型高温超导材料
Manakov方程中调制不稳定性和非简并Akhmediev呼吸子
表面粗糙的聚集和反聚集效应对电子隧穿的巨大影响
对数量子时间晶体
本征微观态的重整化群理论
伊辛模型的几何上临界维度
通过氢化钙退火方法连续掺杂Bi2212至电子掺杂超导体
氦离子注入制备的钇钡铜氧高温超导约瑟夫森结
变分角转移矩阵重正化群方法及其在经典统计模型中的应用
在单比特上基于量子态判别的互文性实验验证
巨行星内部可能存在的氦-二氧化硅化合物
范德瓦尔斯超导材料中准二维超导电性和可调控近藤晶格的共存
QCD强耦合常数在微扰及非微扰能区跑动行为的新分析
始于生成网络的马尔可夫链蒙卡
压力诱导具有超高能量密度的含氖聚合氮化物
FeSe超导体的反常能带劈裂和强各向异性超导能隙随掺硫的奇异演变
锁相热扩散趋肤效应
用于拓扑量子器件的纯相超细InAs–Al纳米线原位分子束外延
α-CsPbI3的缺陷容忍性:高温相材料中点缺陷性质的计算方法
Valence Quark Ratio in the Proton
高混合熵提升非晶合金的能量状态
在可调耦合超导量子比特中实现全微波脉冲的CZ门
BaO/SrTiO3界面的电输运性质研究
高通量第一性原理计算探索潜在的笼目材料

点此浏览所有Express Letters
CPL Express Letters栏目简介
为了保证重要研究成果的首发权和显示度,CPL于2012年6月开设了Express Letters栏目。此栏目发表速度快,学术质量高。截至2020年底,平均每篇被引用约20次,已经在国内物理学界建立起良好口碑与声望,来稿数量不断增加。

https://blog.sciencenet.cn/blog-3426263-1394501.html
上一篇:詹姆斯·韦布太空望远镜的首批科学成果
下一篇:研究快讯 | 基于斯塔克效应实现单个铒离子发光的大范围调谐