关注：

1） 晶体的密排方式；

2） 密排晶体中的四面体间隙和八面体间隙

1. 有时图形胜过文字介绍

http://departments.kings.edu/chemlab/animation/clospack.html

 

网络问答：

AAAA:

体心立方和面心立方的四面体与八面体间隙个数和大小怎么算？

QQQ:

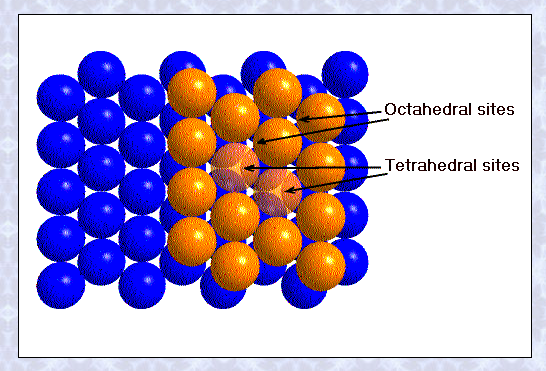
1）四面体空隙：由四个球体围成的空隙，球体中心线围成四面体，

2）八面体空隙：由六个球围成的空隙，球体中心线围成八面体形。

每个球周围都有八个四面体空隙，六个八面体空隙，对有n个等径球体堆积而成的系统，共有：

四面体空隙2n个
八面体空隙n个
证明1）由二维密排球可知，在中心球面上有四个四面体空隙，在下半球面上有四个四面体空隙。

2）由面心立方晶胞图可证明（a）每个球周围有六个八面体空隙，（b）n个球堆积可形成n个八面体空隙， 2n个四面体空隙。

在立方体内有八个四面体空隙，在每条棱中心有一个八面体空隙，在体中心有一个八面体空隙共有 个八面体空隙，面心立方点阵有4个结点。

QQQ::

两种密堆积中，四面体与八面体空隙之比为2：1，八面体空隙数等于原子数。
至于能容纳下的最大原子半径即大小，对于四面体空隙来说，应该用正四面体体心到顶点的距离（即4分之根号6个a，a为四面体边长即堆积原子半径的两倍）减去堆积原子的半径。
对于八面体空隙，两种堆积的算法不一样。
1）体心立方堆积：
由于配位数的关系，将八面体组成中的上面五个原子放到最上面原子的配位立方体中考虑，八面体除上下两个原子外的其余原子组成正方形边长应为三分之四根三倍的原子半径。空隙大小即为正方形对角线长减去原子半径的两倍的差除以二。
2）面心立方堆积：
由于六个原子在晶胞中所处的化学环境一样，所以空隙大小即为根二减1倍的原子半径。

AAA:::

体心立方中原子个数：八面体间隙数：四面体间隙数= ？

QQQ:::

答案应该是（8*1/8+1）:(6*1/2+12*1/4):(12*2*1/2)=1:3:6,

网络摘录：

http://blog.163.com/jiangxunyong@126/blog/static/519977482006297565131/

FCC晶格

BCC晶格

HCP晶格

1） 从表中可以看到BCC结构的四面体和八面体间隙都是不对称的。其棱边长不全相等。

2） FCC和HCP都是密排结构，而BCC则是比较“开放”的结构。其间隙较多。间隙元素在BCC中的扩散速率比在FCC和HCP金属中要高。

3） FCC和HCP金属中的八面体间隙大于四面体间隙，故这些金属中间隙元素的原子必位于八面体间隙中。

4） 在BCC晶体中，四面体间隙大于八面体间隙，因而间隙原子应占据四面体的间隙位置。但是，由于BCC的八面体间隙是不对称的，即使间隙原子占据八面体间隙位置，只会引起距间隙中心为a/2的两个原子显著地偏离平衡位置，其余4个原子则不会显著偏离平衡位置，因而总的点阵畸变不大。因此，在有些BCC金属中，间隙原子占据四面体间隙，而在另一些BCC晶体中，间隙原子占据八面体间隙位置。

5） FCC和HCP中的八面体间隙远大于BCC中的八面体或四面体间隙，因而间隙元素在FCC和HCP中的溶解度往往比在BCC中大的多。

6）FCC和HCP的八面体及四面体间隙大小彼此相等。这是由于其原子堆垛形式很类似。

2. Tetrahedral and Octahedral sites in closest packing

http://www.science.uwaterloo.ca/~cchieh/cact/c123/tetrahed.html

Tetrahedral and Octahedral Sites in Closest PackingSkills to develop

• To construct a model for the tetrahedral and octahedral holes of closest packing

• To calculate geometric properties of the model

• Apply these properties to interpret crystal chemistry

Tetrahedral and Octahedral Sites in Closest PackingTetrahedral and octahedral sites in closest packing can be occupied by other atoms or ions in crystal structures of salts and alloys. Thus, recognizing their existence and their geometrical constrains help the study and interpretation of crystal chemistry. The packing of spheres and the formation of tetrahedral and octahedral sites or holes are shown below.

Whenever you put four (4) spheres together touching each other, you've got a tetrahedral arrangement of spheres. The space in the center is called a tetrahedral site. The octahedral site is formed by six spheres. These sites are also called holes in some literature, and they are shown in the diagrams above.

Example 1

What is the radius of the largest sphere that can be placed in a tetrahedral hole without pushing the spheres apart?

Suggestion for solution
To solve a problem of this type, we need to construct a model for the analysis. The following statement explicitly tells you how to construct such a model.

Use the diagram shown here as a starting point, and construct a tetrahedral arrangement by placing four spheres of radius R at alternate corners of a cube. Once complete, work out the followin:

• What is the length of the face diagonal fd of this cube in terms of R?
  Since the spheres are in contact at the centre of each cube face, fd = 2 R.

• What is the length of the edge for such a cube, in terms of R?
  Cube edge length a = Ö2 R
  

• What is the length of the body diagonal bd of the cube in R?
  bd = Ö6 R

• Is the center of the cube also the center of the tetrahedral hole?
  Yes, but do you know why?

• Let the radius of the tetrahedral hole be r, express bd in terms of R and r
  If you put a small ball there, it will be in contact with all four spheres. Thus,

  bd = 2 (R + r). r = (2.45 R) / 2 - R
     = 1.225 R - R
     = 0.225 R
  

  
  

• 
  

• What is the radius ratio of tetrahedral holes to the spheres?
  

  r / R = 0.225

  
  

Example 2

What is the radius of the largest sphere that can be placed in an octahedral hole without pushing the spheres apart?

Solution
The octahedral hole is located at the center of any four spheres that form a square. If we represent the radius of a ball fitting in the octahedral holes byr, and the radius of the sphere as R, then we have the relationship:

r + R = (¹/_Ö2) (2 R)
^r / _R = Ö2 - 1
     = 0.414

The implication:
Pure geometric consideration shows that only small balls fit in the tetrahedral holes of packed spheres. However, if the radii of cations are smaller than 0.225R, the structure of having ions in the tetrahedral site is unstable. The anions may be pushed apart slightly to reduce the repulsion by fitting a cation in the tetrahedral site.

For ionic crystal structure consideration, the cations are usually smaller than anions. Cations fitting into the tetrahedral sites cannot be smaller than 0.225 R. Usually, most ions are slightly larger than 0.225 R, but smaller than 0.414 R. In such cases, the cation coordination is tetrahedral, and a typical structure is ZnS, although covalent bonding is also involved in ZnS. The animated diagram is a model of ZnS structure.

When the cation radii are greater or equal to 0.414 R, but less than 0.732 R, the cations occupy the octahedral sites. Sodium chloride is one such structure, and it serves as an important structure type.

If the cations are large such that r > 0.732 R, the cation will have a cubic coordination of 8. The strcture is typified by CsCl.

The above discussion is summarized below:

r/R	0.225	between	0.414	between	0.732	<
Coordination & number	"	tetrahedral 4	"	octahedral 8	"	cubic 8
Typical structure	"	ZnS	"	NaCl	"	CsCl

For an interesting, illustrateive and exciting discussion regarding radius ratio and types of inorganic solids, see Structures of Simple Inorganic Solids by Dr. S.J. Heyes. This link is aimed at a higher level than that of a first year chemistry course, but the content is great.

Discussion
What is the radius of the largest sphere that can be placed in an octahedral hole without pushing the spheres apart?
(Answer: 0.731)

Example 3

Is there a structure in which all the tetrahedral sites are occupied by a different type of atoms or ions?

Solution
The outline of a unit cell for PuO₂ is shown here, and all the tetrahedral sites are occupied by small O^2- ions.

Actually, the crystal structure of UO₂ has the same structure as PuO₂. A common salt CaF₂ also has the same structure, but the fluoride ions are by no means small compared to the calcium ions. However, the Pu⁴⁺ and U⁴⁺ ions are large compared to the oxygen ions.

Confidence Building Questions

• In the NaCl structure, which ion is larger?

     Hint . . .    The chloride ion is larger.

  Discussion -
  Usually anions are larger than cations if they have similar electronic configurations. In this case, the Na⁺and Cla^- ions have electronic configurations of Ne and Ar respectively. We already know that Ar is larger than Ne.

  
  

  
  

• The ionic radii of Na⁺ and Cl^- ions are 91 and 181 pm respectively. Calculate the radii ratio of cation to anions.

     Hint . . .    91/181 = 0.5

  Discussion -
  Since the ratio of 0.5 is between 0.414 and 0.732, the sodium ion prefers an octahedral coordination.

  
  

  
  

• The ionic radii of K⁺ and I^- ions are 133 and 220 pm respectively. What type of structure should KI have?

     Hint . . .    The ratio = 133/220 = 0.605, the NaCl structure type prevail.

  Discussion -
  Structure of KI is indeed NaCl type, with a = 706 pm. The data agree with the ionic radii of the ions 706 = 2 (133 + 220).

  
  

  
  

• The ionic radii of Cs⁺ and Cl^- ions are 167 and 181 pm respectively. What type of coordination should Cs⁺ have?

     Hint . . .    The ratio = 167/181 = 0.923, Cs ion has cubic coordination.

  Discussion -
  The CsCl structure is a an important type of structures. The diagram show a unit cell of the CsCl structure.

  
  

转载本文请联系原作者获取授权，同时请注明本文来自叶小球科学网博客。
链接地址：https://blog.sciencenet.cn/blog-567091-738778.html

上一篇：感悟之：review report与writing a paper
下一篇：Build intuition之: 常见晶体结构类型再谈