“人是社会关系的总和，人不能脱离社会而存在。”

物质世界是“普遍联系”和“永恒发展”的。

数学是现实世界中的“数量关系”和“空间形式”的科学。

真理越辩越明。

汉语是联合国官方正式使用的 6 种同等有效语言之一。请不要歧视汉语！

Chinese is one of the six equally effective official languages of the United Nations.

Not to discriminate against Chinese, please!

[请教，讨论] P对NP（九）：请您看看，您还有哪些批评或疑问？

一、“P对NP”，共计“1+3”个角度

   从集合论，

   从“立体图”，

   从“无穷”，

   从“概率”，

   共计“1+3”个角度，反思几十年了。我实在看不出还有什么漏洞。

   “P对NP”问题，

   不仅仅从“集合论”角度，

   并且从

   “形状、数量”、“有穷、无穷”、“确定、概率”这三种不同角度

   都思考过了。

   一个问题，“1+3”类数学角度思考，是不是已经前无古人了？

   算不算

   从“源头和底层”完整地解决了“P对NP”？

   由 ZF 里的“幂集公理”可以发现：NTM 相当于 DTM 的幂集。

   According to "the axiom of power set" in ZF, a NTM is equipotent to the power set of its corresponding DTM.

图1  “P对NP”，共计“1+3”个角度

二、“P对NP”的3个直接后果

   演绎证明的实质；完全证明。

   第二类数域。

   人脑复杂性估计。

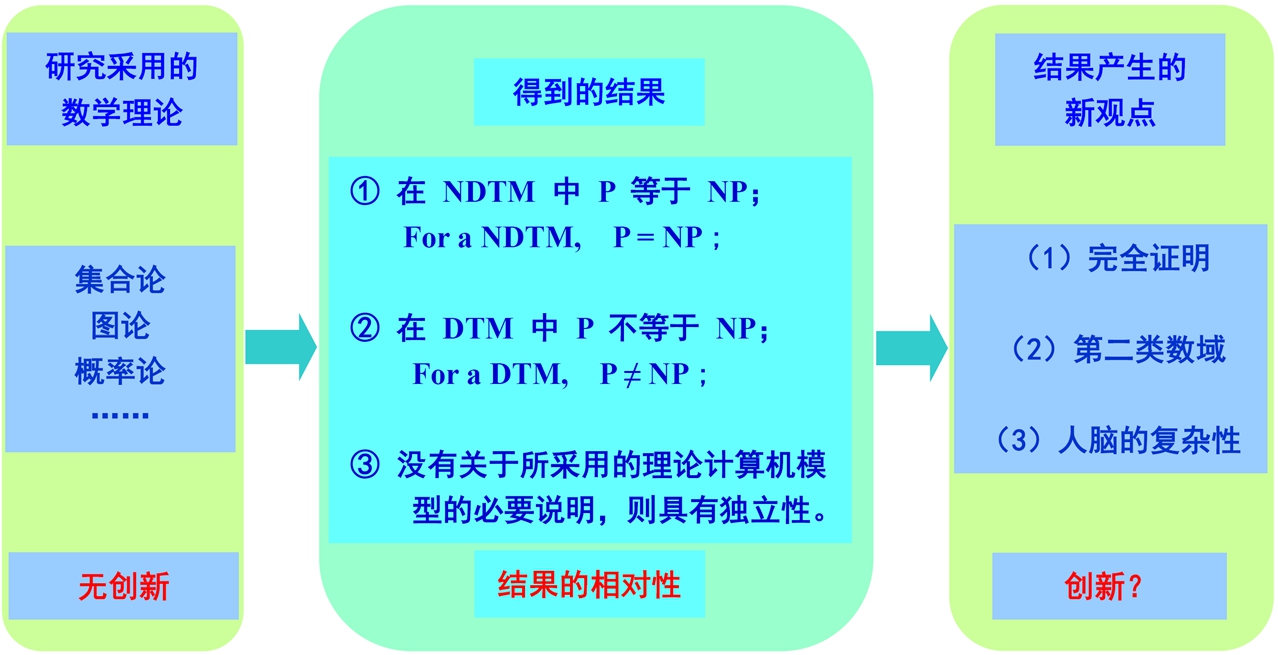
图2  “P对NP”的3个直接后果。其实还有更多的后续问题待研究。

三、您还有哪些批评或疑问？

   敬请批评指正！

   感谢！

   假如您一时没有不同意见，

   我

   就去做别的事情了。

   例如“磁场、电磁波依赖参照系”的判定实验原理。

   不仅人类历史上物理学家综合排行榜第二的爱因斯坦强调实验比逻辑更重要，俺的偶像爱因斯坦（Albert Einstein）1933-06-10 在the Herbert Spencer lecture at Oxford 《On the Method of Theoretical Physics 关于理论物理学的方法》里说: “Pure logical thinking cannot yield us any knowledge of the empirical world; all knowledge of reality starts from experience and ends in it. Propositions arrived at purely by logical means are completely empty as regards reality. Because Galileo saw this, and particularly because he drummed it into the scientific world, he is the father of physics —— indeed of modern science altogether. 纯粹的逻辑思维不能给我们任何关于经验世界的知识；一切关于实在的知识，都是从经验开始，又终结于经验。就现实而言，纯粹通过逻辑手段得出的主张是完全空白的。由于伽利略看到了这一点，特别是因为他将其强力引入了科学界，因此他是物理学的父亲 —— 完全代表了现代科学。”

   就连人类历史上数学家综合排行榜第三的庞加莱也强调逻辑的局限性。

图3  （美）玛莎·葛森著. 完美的证明 一位天才和世纪数学的突破[M]. 2012 page 153 截图

   网传庞加莱公然说：逻辑是证明的工具，直觉是发明的工具。逻辑可以告诉我们走这条路或那条路保证不遇见任何障碍，但是它不能告诉我们哪条道路能引导我们到达目的地。为此必须从远处瞭望目标，教导我们瞭望的本领的是直觉。没有直觉，数学家就会像这样一个作家，他只会按语法写诗，但是却毫无思想。

   我只找到了“It is by logic we prove, it is by intuition that we invent. 我们用逻辑证明，用直觉发明。”，以及“Logic, therefore, remains barren unless fertilised by intuition. 因此，除非得到直觉的滋养，否则逻辑仍然是贫瘠的。”

https://mathshistory.st-andrews.ac.uk/Biographies/Poincare/

   当别人问高斯如何得到他定理，人类历史上数学家综合排行榜第二的高斯，毫不含糊地说：“…通过系统的、明显的实验。... through systematic, palpable experimentation. ... durch planmässiges Tattonieren.”：

https://mathshistory.st-andrews.ac.uk/Biographies/Gauss/quotations/

   伟大数学家高斯在1830年给贝塞尔的信中写道：

   "We must admit with humility that, while number is purely a product of our minds, space has a reality outside our minds, so that we cannot completely prescribe its properties a priori. 我们必须谦虚地承认，虽然数字纯粹是我们头脑的产物，但空间在我们头脑之外有一个现实，因此我们不能完全先验地规定其性质。"

https://mathshistory.st-andrews.ac.uk/Biographies/Gauss/quotations/

   人类历史上一位真正的大专家一生爱书如命，反映出他的深远视野和超凡气魄。他多次向青年人和他的亲近者强调，读书对于人的成长和视野的开阔有着不可估量的作用，他更推荐大家多读一些被称为“闲书”或者“散书”的书籍。

   大专家这样的建议源于他的深思熟虑。“他深知人的精力和视野是有限的，多年的生活和工作经历会让人的视野变得狭窄。因此，他强调阅读，因为阅读可以让我们突破这些限制，开阔视野，看到更多的世界。”

   至于人类历史上数学家综合排行榜第一的柯西为什么没有在逻辑局限性方面表态？首先，可能是我还没有阅读到。其次，更可能是因为柯西忙于各种具体数学问题的研究，没有精力了。

   柯西晚年恶心伽罗华（Evariste Galois）、阿贝尔（Niels Henrik Abel），不排除柯西“被”要求评论的可能性。可能此时柯西已经太累了。没有精力去评审了。

   至于人类历史上物理学家综合排行榜第一的牛顿么，网传他说：“没有大胆的猜测就作不出伟大的发现。”

参考资料：

[1] 2022-07-13，策梅洛-弗兰克尔集合论/Zermelo-Fraenkel set theory/杜国平，中国大百科全书，第三版网络版[DB/OL]

https://www.zgbk.com/ecph/words?SiteID=1&ID=229361&Type=bkzyb&SubID=104156

[2] 2023-01-18，数学基础/foundations of mathematics/何浩平，中国大百科全书，第三版网络版[DB/OL]

https://www.zgbk.com/ecph/words?SiteID=1&ID=456822&Type=bkzyb&SubID=137849

   同时发展出的公理集合论成为了事实上的数学基础。

   20世纪初数学基础研究的结果，是数学家们在实践中接受了公理化的集合论作为经典数学的基础。

   E.F.F. 策梅洛、A.A.弗伦克尔（Adolf Abraham Fraenkel）等人发展出的ZFC（策梅洛-弗伦克尔-选择公理）公理集合论，是今天通行的公理集合论。ZFC公理集合论是万有理论，能够推导出经典数学的所有理论。

[3] ZFC. Encyclopedia of Mathematics.   Zermelo–Fraenkel set theory with the axiom of choice

https://encyclopediaofmath.org/wiki/ZFC

一些近年的参考文献：

[1] Lance Fortnow. Fifty years of P vs. NP and the possibility of the impossible [J]. Communications of the ACM, 2022, 65(1): 76–85.

doi:  10.1145/3460351

https://m-cacm.acm.org/magazines/2022/1/257448-fifty-years-of-p-vs-np-and-the-possibility-of-the-impossible/fulltext?mobile=true

[2] Jorge A. Ruiz-Vanoye, Ocotlán Díaz-Parra, Francisco Rafael Trejo-Macotela, Julio Cesar Ramos-Fernández. Editorial: P versus NP problem from formal languages theory view [J]. International Journal of Combinatorial Optimization Problems and Informatics, 2021, 12(1): 1-8. Jan-April 2021

https://webdesignwaterloo.net/all-p-problems-are-np-problesm-scholarly-articles

[3] Luciana S. Buriol, Celina Figueiredo, Mauricio G. C. Resende, Eduardo Uchoa. The guide to NP-completeness is 40 years old: an homage to David s. Johnson [J]. Pesquisa Operacional, 2020, 40: e236329, 1-11

doi:  10.1590/0101-7438.2020.040.00236329

https://www.scielo.br/j/pope/a/Cw4K8K374SySpJdNdx9kPLx/?lang=en

[4] Juraj Hromkoviˇc, Peter Rossmanith. What one has to know when attacking P vs. NP [J]. Journal of Computer and System Sciences, 2020, 107: 142-155. February 2020

doi:  10.1016/j.jcss.2019.06.004

https://www.sciencedirect.com/science/article/pii/S0022000019300558

[5] Igor L. Markov. Limits on fundamental limits to computation [J]. Nature, 2014, 512((7513): 147-154.

doi:  10.1038/nature13570

https://www.nature.com/articles/nature13570

[6] Lance Fortnow. The Status of the P Versus NP Problem [J]. Communications of the ACM, 2009, 52(9): 78-86. 

doi:  10.1145/1562164.1562186 

https://m-cacm.acm.org/magazines/2009/9/38904-the-status-of-the-p-versus-np-problem/fulltext

发表的论文：

[1] A non-canonical example to support that P is not equal to NP. Transactions of Tianjin University, 2011, 17(6): 446-449.

doi:  10.1007/s12209-011-1593-5

https://link.springer.com/article/10.1007/s12209-011-1593-5 

[2] 第二类计算机构想. 中国电子科学研究院学报, 2011, 6(4): 368-374.

doi:  10.3969/j.issn.1673-5692.2011.04.009

https://d.wanfangdata.com.cn/periodical/dzkxjspl201104009

[3] 密码学与非确定型图灵机. 中国电子科学研究院学报, 2008, 3(6): 558-562.

http://qikan.cqvip.com/Qikan/Article/Detail?id=28856183&from=Qikan_Search_Index

[4] 人脑有多复杂？[J]. 百科知识, 1997, 7(总第216期): 39-40.

https://www.cnki.com.cn/Article/CJFDTotal-BKZS199707022.htm

[5] 人类智能模拟的“第2类数学（智能数学）”方法的哲学研究 [J]. 哲学研究, 1999, (4): 44-50.

https://www.cnki.com.cn/Article/CJFDTOTAL-ZXYJ199904005.htm

相关链接：

[1] 2023-07-09，[请教，讨论] P对NP（八）：“P对NP”为什么这么难？

https://blog.sciencenet.cn/blog-107667-1394724.html

[2] 2023-07-08，[请教，讨论] P对NP（七）：“形转换”与立体图、平面图

https://blog.sciencenet.cn/blog-107667-1394594.html

[3] 2023-07-07，[请教，讨论] P对NP（六）：无穷化版本与连续统假设CH

https://blog.sciencenet.cn/blog-107667-1394463.html

[4] 2023-07-05，[讨论] P对NP（五）：宇宙“热寂”之前，“幂集公理”不会有太大的毛病？

https://blog.sciencenet.cn/blog-107667-1394169.html

[5] 2023-07-04，[请教] P对NP（四）：相关要点小结（问答式）

https://blog.sciencenet.cn/blog-107667-1394027.html

[6] 2023-06-29，[请教] P对NP（三）：“NP完全性, NP-completeness”之后

https://blog.sciencenet.cn/blog-107667-1393466.html

[7] 2022-06-10，[请教] P对NP（二）：结果的相对性与“1+3”种证明

https://blog.sciencenet.cn/blog-107667-1342404.html

[8] 2012-03-23，[请教] P对NP：请***教授等专家指教（一）

https://blog.sciencenet.cn/blog-107667-550859.html

[9] 2023-01-16，[搞笑？搞哭？汇集] 怎样判断“原创”和“诺贝尔奖成果”？

https://blog.sciencenet.cn/blog-107667-1372203.html

[10] 2015-05-22，The kernel of "P vs NP Problem": Axiom of power set!

https://blog.sciencenet.cn/blog-107667-892400.html

感谢您的指教！

感谢您指正以上任何错误！

感谢您提供更多的相关资料！