注：

1)   Pu: d-eg  与d-t2g的区别

2)   4f 与5f电子的区别

3） 什么是LSDA(Local  spin density and generalized  gradient)?  

4)   什么是LSDA+U

5)  电子相关---造成电子局域，并阻止5f电子成键

6）  怎样从态密度图或电荷密度图，看出共价键和金属键的区别

7）Mott-Hubbard绝缘体 or  

  In general, insulators can be divided into Mott-Hubbard type insulators and charge transfer insulators.

8） Pierrs 转变及焦汤转变

9）  Hubbard   parameter  U

10)   Tight-binding model

12)    Mott insulators

13） 什么是spin corssover? 高压下，5f电子的离域定域关系会发生什么样的变化？  spin corssover呢？

spin crossover

1. Wikipeida:  Hubbard parameter

http://en.wikipedia.org/wiki/Hubbard_model

The Hubbard model is an approximate model used, especially in solid state physics, to describe the transition between conducting and insulating systems.^[1] The Hubbard model, named after John Hubbard, is the simplest model of interacting particles in a lattice, with only two terms in the Hamiltonian (see example below): a kinetic term allowing for tunneling ('hopping') of particles between sites of the lattice and a potential term consisting of an on-site interaction.

     The particles can either be fermions, as in Hubbard's original work, or bosons, when the model is referred to as either the 'Bose–Hubbard model' or the 'boson Hubbard model'.

 The Hubbard model is a good approximation for particles in a periodic potential at sufficiently low temperatures that all the particles are in the lowest Bloch band, as long as any long-range interactions between the particles can be ignored.        

   If interactions between particles on different sites of the lattice are included, the model is often referred to as the 'extended Hubbard model'.

The model was originally proposed (in 1963) to describe electrons in solids and has since been the focus of particular interest as a model for high-temperature superconductivity. More recently, the Bose-Hubbard model has been used to describe the behavior of ultracold atoms trapped in optical lattices. Recent ultracold atom experiments have also realised the original, fermionic Hubbard model in the hope that such experiments could yield its phase diagram.^[2]

    For electrons in a solid, the Hubbard model can be considered as an improvement on the tight-binding model, which includes only the hopping term(跳跃；).

  For strong interactions, it can give qualitatively different behavior from the tight-binding model, and correctly predicts the existence of so-called Mott insulators, which are prevented from becoming conducting by the strong repulsion between the particles.

• 1Theory (Narrow energy band theory)

• 2Example: 1D chain of hydrogen atoms

• 3More complex systems

• 4Numerical treatment

• 5See also

• 6References

• 7Further reading

• 8External links

Theory

The Hubbard model is based on the tight-binding approximation from solid state physics. In the tight-binding approximation, electrons are viewed as occupying the standard orbitals of their constituent atoms, and then 'hopping' between atoms during conduction.

  Mathematically, this is represented as a 'hopping integral' or 'transfer integral' between neighboring atoms, which can be viewed as the physical principle that creates electron bands in crystalline materials, due to overlapping between atomic orbitals.

The width of the band depends upon the overlapping amplitude（幅度）. However, the more general band theories do not consider interactions between electrons explicitly. They consider the interaction of a single electron with the potential of nuclei and other electrons in an average way only. By formulating conduction in terms of the hopping integral, however, the Hubbard model is able to include the so-called 'onsite repulsion', which stems from the Coulomb repulsion between electrons at the same atomic orbitals. 【来自同一原子轨道中电子之间的相互排斥作用】

This sets up a competition between the hopping integral, which is a function of the distance and angles between neighboring atoms, and the on-site Coulomb repulsion, which is not considered in the usual band theories.

    The Hubbard model can therefore explain the transition from metal to insulator in certain transition metal oxides as they are heated by the increase in nearest neighbor spacing, which reduces the 'hopping integral' to the point where the onsite potential is dominant.

     Similarly, this can explain the transition from conductor to insulator in systems such as rare-earth  pyrochlores as the atomic number of the rare-earth metal increases, because the lattice parameter increases (or the angle between atoms can also change — see Crystal structure) as the rare-earth element atomic number increases, thus changing the relative importance of the hopping integral compared to the onsite repulsion.

Example: 1D chain of  hydrogen atoms

The hydrogen atom has only one electron, in the so-called s orbital, which can either be spin up () or spin down (). This orbital can be occupied by at most two electrons, one with spin up and one down (see Pauli exclusion principle).

Now, consider a 1D chain of hydrogen atoms.

Under band theory, we would expect the 1s orbital to form a continuous band, which would be exactly half-full.

The 1-D chain of hydrogen atoms is thus predicted to be a conductor under conventional band theory.

But now consider the case where the spacing between the hydrogen atoms is gradually increased. At some point we expect that the chain must become an insulator.

Expressed in terms of the Hubbard model, on the other hand, the Hamiltonian is now made up of two components.

     The first component is the hopping integral【积分】. The hopping integral is typically represented by the letter t because it represents the kinetic energy of electrons hopping between atoms.

The second term in the Hubbard model is then the on-site repulsion, typically represented by the letter U because it represents the potential energy arising from the charges on the electrons. Written out in second quantization notation【符号】, the Hubbard Hamiltonian then takes the form:

where represents nearest-neighbor interaction on the lattice.

If we consider the Hamiltonian without the contribution of the second term, we are simply left with the tight binding formula from regular band theory.

When the second term is included, however, we end up with a more realistic model that also predicts a transition from conductor to insulator as the interatomic spacing is increased.    

In the limit where the spacing is infinite (or if we ignore the first term), the chain simply resolves into a set of isolated magnetic moments.

 Additionally, when there are some contributions from the first term, but the material remains an insulator, the overlap integral provides for exchange interactions between neighboring magnetic moments which may lead to a variety of interesting magnetic correlations, such as ferromagnetic, antiferromagnetic, etc. depending on the exact solutions of the model. The one dimensional Hubbard model was solved by Lieb and Wu using the Bethe ansatz. Essential progress has been achieved in the 1990s: a hidden symmetry was discovered, the scattering matrix, correlation functions, thermodynamic and quantum entanglement were evaluated, see.^[3]

More complex systems

Although the Hubbard model is useful in describing systems such as a 1-D chain of hydrogen atoms, it is important to note that in more complex systems there may be other effects that the Hubbard model does not consider. In general, insulators can be divided into Mott-Hubbard type insulators and charge transfer insulators.

Consider the following description of a Mott-Hubbard insulator:

(Ni²⁺O^2-)₂ --> Ni³⁺O^2- + Ni¹⁺O^2-

This can be seen as analogous to the Hubbard model for hydrogen chains, where conduction between unit cells can be described by a transfer integral.

However, it is possible for the electrons to exhibit another kind of behavior:

Ni²⁺O^2- --> Ni¹⁺O^1- 【为什么称这种情形为  charge transfer insulator?  不懂】

This is known as charge transfer, and results in charge transfer insulators. Note that this is quite different from the Mott-Hubbard insulator model because there is no electron transfer between unit cells, only within a unit cell.

Both of these effects may be present and competing in complex ionic systems.

Numerical treatment

The fact that the Hubbard model cannot be solved analytically in arbitrary dimensions has led to intense research into numerical methods for these strongly correlated electron systems.^[4]

Exact treatment of the Hubbard model at absolute zero is possible using the Lanczos algorithm, which produces static as well as dynamic properties of the system. This method requires the storing of three vectors of the size of the number of states, which limits the number of sites in the lattice to about 20 on currently^[when?] available hardware. With projector and finite-temperature auxiliary field Monte Carlo two statistical methods exist that also can provide an exact solution. For low temperatures and large lattice sizes convergence problems appear that lead to an exponential growth of computational effort due to the so-called sign problem. The Hubbard model can also be studied within dynamical mean field theory (DMFT). This scheme maps the Hubbard Hamiltonian onto a single site impurity model, which allows one to compute the local Green's function of the Hubbard model for a given and a given temperature. Within DMFT, one can compute the evolution of the spectral function and observe the appearance of the upper and lower Hubbard bands as correlations increase.

See also

• Bloch wave

• Electronic band structure

• Solid state physics

• Bose–Hubbard model

• t-J model

• Dynamical Mean Field Theory

Wikipedia中文解释

赫巴德模型[编辑]

莫特绝缘体[编辑]

维基百科，自由的百科全书

（重定向自莫特绝缘体）

跳转至：导航、搜索

莫特绝缘体的二维相。

莫特绝缘体〈玻色气体〉的二维原子相变。

莫特绝缘体〈英语：Mott insulator〉的命名是为纪念英国物理学家兼1977年诺贝尔物理奖得主内维尔·莫特。

莫特绝缘体是应该分类在常规能带理论之下的导体，当在特别低温测量时是绝缘体。这个作用归结于电子和电子的相互作用，在常规能带理论上没有被考虑。

虽然固体的能带理论是非常成功的在描述材料电子特性。但在1937年时，Jan Hendrik de Boer（英语：Jan Hendrik de Boer）和 Evert Johannes Willem Verwey即已指出不同的过渡金属氧化物可以被能带理论预测是导体(因为在每单位晶格有奇数个电子)或是绝缘体。内维尔·莫特和Rudolf Peierls也在1937年然后预言这个反常现象可以被解释用包括电子之间的互作用。

wikipedia 英文解释

Mott insulators are a class of materials that should conductelectricity under conventional band theories, but are insulators when measured (particularly at low temperatures). This effect is due to electron–electron interactions, which are not considered in conventional band theory.

The bandgap in a Mott insulator exists between bands of like character, such as 3d character, whereas the bandgap in charge transfer insulators exists between anion and cation states (see lecture slides ), such as between O 2p and Ni 3d bands in NiO. ^[1]

History[edit]

Although the band theory of solids had been very successful in describing various electrical properties of materials, in 1937 Jan Hendrik de Boer and Evert Johannes Willem Verwey pointed out that a variety of transition metal oxides predicted to be conductors by band theory (because they have an odd number of electrons per unit cell) are insulators.^[2]Nevill Mott and Rudolf Peierls then (also in 1937) predicted that this anomaly can be explained by including interactions between electrons.^[3]

In 1949, in particular, Mott proposed a model for NiO as an insulator, where conduction is based on the formula^[4]

(Ni²⁺O²⁻)₂ → Ni³⁺O²⁻ + Ni¹⁺O²⁻.

In this situation, the formation of an energy gap preventing conduction can be understood as the competition between the Coulomb potentialU between 3d electrons and the transfer integral t of 3d electrons between neighboring atoms (the transfer integral is a part of the tight-binding approximation). The total energy gap is then

E_gap = U − 2zt,

where z is the number of nearest-neighbor atoms.

In general, Mott insulators occur when the repulsive Coulomb potential U is large enough to create an energy gap. One of the simplest theories of Mott insulators is the 1963 Hubbard model. The crossover from a metal to a Mott insulator as U is increased can be predicted within the so-called Dynamical Mean Field Theory.

Mottness[edit]

Mottism denotes the additional ingredient, aside from antiferromagnetic ordering, which is necessary to fully describe a Mott Insulator. In other words, we might write

antiferromagnetic order + mottism = Mott insulator

Thus, mottism accounts for all of the properties of Mott insulators that cannot be attributed simply to antiferromagnetism.

There are a number of properties of Mott insulators, derived from both experimental and theoretical observations, which cannot be attributed to antiferromagnetic ordering and thus constitute mottism. These properties include

• Spectral weight transfer on the Mott scale ^[5][6]

• Vanishing of the single particle Green function along a connected surface in momentum space in the first Brillouin zone^[7]

• Two sign changes of the Hall coefficient as electron doping goes from 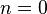to (band insulators have only one sign change at )

• The presence of a charge (with the charge of an electron) boson at low energies ^[8][9]

• A pseudogap away from half-filling () ^[10]

Applications[edit]

Mott insulators are of growing interest in advanced physics research, and are not yet fully understood. They have applications in thin-filmmagneticheterostructures and high-temperature superconductivity, for example.^[11]

This kind of insulator can become a conductor if an external voltage is applied across the material. The effect is known as a Mott transition and can be used to build smaller field-effect transistors, switches and memory devices than possible with conventional materials.^[12]

网络资料查询：

1.  经常在文献中看见电荷密度图和差分电荷密度图，还有p轨道，d轨道什么的。
p、d轨道的结论是从电荷密度图分析得到的？还是从差分电荷密度图得到的。
什么样的图形可以解释为p轨道，什么样的图形可以解释为d轨道啊？
d轨道分为d(eg)和d(t2g)，p轨道分为px，py，pz。
d(eg)和d(t2g)是什么意思啊？
d(eg)，d(t2g)，px，py，pz能用vasp计算吗？

在图中怎么判断d轨道是d(eg)轨道，还是d(t2g)轨道？

AAAA:::

1. p轨道是哑铃型的，d轨道是花瓣型的。
    通常的p轨道和d轨道可以看作是氢原子的轨道角动量为1和2的电子轨道（也可以是其它类型的轨道，如Gaussian型轨道等）。所

    所谓的px,py,pz和坐标系的选取有关；轨道角动量为1（2）的（氢）原子轨道有三（五）个，px,py,pz是其中的一种选取方法，还有一种选法是p1,p0,p-1。d轨道的情况类似。
2. 所谓的电荷密度图应该是某一分子轨道（通常为前线轨道）的电子云分布情况；从该分子轨道的（LCAO）展开系数可以看出对该分子作重要贡献的原子轨道（如这里的p或者d轨道）。
3. eg,t2g是相应原子或分子轨道所属的不可约表示，和分子以及分子轨道的对称性有关。

4. 如1所述，p轨道和d轨道都是确定的函数，几乎所有的量化软件里都有相关信息，不用计算。

1. Quantum Chemistry (6th Edition), Ira N. Levine, Prentice Hall, 2008.
2. Ideas of Quantum Chemistry, Lucjan Piela, Elsevier, 2007.

PS: 书太多了，随便推荐两本。 Levine的量子化学是经典著作；这两本书网上都有电子版。还有一些中文版的量子化学书，不过真心不推荐

QQQ：：：：

MS中的问题，不太明白Hubbard U与U、J的意义，J值如何得到？麻烦高人指点下！
有资料说：“Materials Studio 4.3版本中也给出了一些元素默认的U数值（实际是Ueff＝U－J）”还是不大明白

J. Phys.: Condens. Matter 15 (2003) 979–996

本人基础较差！:rol:

AAA：：：
The Coulomb repulsion is characterized by a spherically averaged Hubbard parameter
U describing the energy increase for placing an extra electron on a particular site, U = E(dn+1) + E(dn−1) − 2E(dn), and a parameter J representing the screened exchange energy.
While U depends on the spatial extent of the wavefunctions and on screening, J is an
approximation of the Stoner exchange parameter and almost constant, ∼1 eV .

    The
Mott–Hubbard Hamiltonian includes energy contributions already accounted for by the DFT
functional. To correct for this ‘double-counting’, equation (1) is evaluated in the limit of integer
occupancies and subtracted from the DFT energy to obtain the spin-polarizedDFT+U energy
functional . A simple functional is obtained after some straightforward algebra