博文
What is Mathematical Game Theory (#2)
 精选
精选
|
(For new reader and those who request 好友请求, please read my 公告栏 first).
I now continue with my example from
Using the illustration of the Arm Race interpretation, we see that the Nash Equilibrium is not always a desirable solution. No one dares to unilaterally deviate from the equilibrium solution. We can in fact see this very clearly in Fig.5 (amplified, simplified, and stylized in Fig. 6) below:

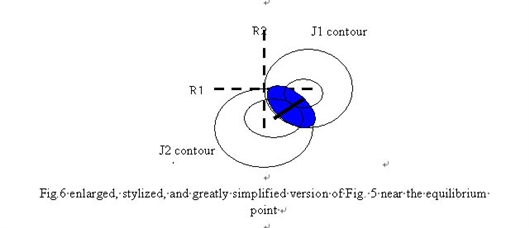
The blue shaded region next to the equilibrium point is the so-called “Prisoner’s Dilemma Region (Note if you Google or Wikipedia the name you will get a full explanation of the origin of the name. Note added 4/13/08 In the April 2008 issue of Scientific American there is an article about the inevitability of cheating in sports such a cycling. The article use the example of Prisoner's Dilemma to explain the reasoning behind the phenomunum ) Here we shall call it the Arms Trace Dilemma solution region. In this lense shaped area, every point is a better solution point for BOTH the US and USSR since every point is at a higher elevation than the Nash point. The only problem is that to reach such a point requires COOPERATION by both decision makers. For example if DM2 unilaterally moves to the right along the θ2 axis, hoping to move into the region and thus resulting in a higher elevation, but DM1 does not reciprocate by also decrease θ1, then DM2 will suffer. In such a game situation, to achieve cooperation you need external means to enforce it. For example, during the Cold War, the US and USSR signed the SALT (Strategic Arms Limitation Treaty) agreement.
If both decision makers decide to cooperate, there are still infinite number of points in the blue shaded region they could choose. Which point should they agree on? Clearly they should avoid points that are dominated by other points (in the sense that there exist other points that are better for BOTH decision makers.). This consideration results in what is known as the Contract Curve. This is the locus of points which are the tangent points between the contours of DM1 and DM2. We indicate this by the line (curve) in Fig.6.
Contract curve means that if the two side is going to sign a contract of agreement or treaty, then they only need to consider points along this curve. This is because no point on this curve is dominated by another point. In other words, if you move away from any points on this curve, at least one side will suffer and both side cannot improve.
Look at the problem another way, once the two decision maker decides to cooperative, they might as well entrust they joined decision to a super decision maker who will choose both θ1 and θ2 with a vector objective of J1 and J2. In vector optimization, the corresponding concept of maximum or minimum is called “pareto optimality”. Conceptually, suppose we consider a thought experiment during which we try out all possible combinations of the decision makers choice θ1 and θ2. This will result in all possible realizations of the objectives J1 and J2. If we plot these points in the J1-J2 plane, This constitutes the set of reachable objectives by the two cooperating decision makers. From the view point of vector maximization, the best possible realizations are the north east boundary of this reachable set (assuming both DM1 and DM2 are maximizing). They are the points not dominated by other reachable points. And the contract curve must be part of this north-east boundary (or pareto frontier).
Pareto optimality for vector optimization is the analog of maximum or minimum in single objective optimization. We illustrate this is Figure 7.

A number of concepts were introduced in Figures 5-7. Readers should stop and think this through before I go on.
(NOTE: My ability to compose graphics in WORD document is limited. It took me a lot of time to achieve imperfect and sometimes inaccurate figures and drawings. My apologies to careful readers who may see such imperfections)
https://blog.sciencenet.cn/blog-1565-20337.html
上一篇:能吃苦的好理由 From the Op-Ed pages of NYT Apr.2, 2008
下一篇:Key US Science and Engineering Indicators 2008