博文
胜过 Fisher z 变换!(1)
|||
胜过 Fisher z 变换!(1)
关键词:数理统计学,相关系数,置信区间,正态分布,累积分布函数,Fisher z transformation,初等函数,显式,高斯误差函数,非初等函数.
我们发现了两个新的初等显式函数,在逼近标准正态分布累积分布函数时,误差小于著名的 Fisher z 变换。
Fisher z 变换(Fisher z-transform,Fisher z transformation,Fisher's z' transformation,Fisher's Z Transformation)在当前国内外数理统计学教材中普遍使用。Fisher在1915年提出该变换。
其中的一个新函数 Quadratic Radical Function,已经被我们学校的TTU(Transactions of Tianjin University)录用。预期在2013年下半年刊出。
另外一个新函数还在争取发表的过程中。
TTU录用新函数逼近标准正态分布累积分布函数的误差如下图。其中红色细线为 Fisher z 变换的逼近误差,蓝色细线为我们的新函数。两者优化配合的误差为绿色点线。由于版权等科技规范的限制,这里我们不能提供有关的量化细节,只能提供直观的定性对照。
Quadratic Radical Function 很简单,对改进某重要问题的计算机算法,有很好的作用。

感谢TTU有关专家和领导!
在《[求教] Quadratic radical function 胜过 Fisher Z Transformation 后的困惑》
http://bbs.sciencenet.cn/blog-107667-588530.html
————————— 相关背景 —————————

Sir Ronald Aylmer Fisher
Photograph courtesy of Professor A W F Edwards by kind permission of Joan Fisher Box
http://www.galtoninstitute.org.uk/Newsletters/GINL0306/university_of_cambridge_eugenics.htm
在《大英百科全书,Encyclopaedia Britannica》
http://www.britannica.com/EBchecked/topic/208658/Sir-Ronald-Aylmer-Fisher
Sir Ronald Aylmer Fisher, byname R.A. Fisher (born February 17, 1890, London, England—died July 29, 1962, Adelaide, Australia), British statistician and geneticist who pioneered the application of statistical procedures to the design of scientific experiments.
在《The MacTutor History of Mathematics archive》
http://www-history.mcs.st-andrews.ac.uk/Biographies/Fisher.html
Fisher z-transform 在《苏联数学百科全书》的当前网络版
词条“Correlation (in statistics)”
http://www.encyclopediaofmath.org/index.php/Correlation_(in_statistics)
If  one usually uses the Fisher z-transform, with
one usually uses the Fisher z-transform, with 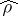 replaced by z according to the formula
replaced by z according to the formula
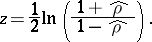 |
Even at relatively small values  the distribution of
the distribution of  is a good approximation to the normal distribution with mathematical expectation
is a good approximation to the normal distribution with mathematical expectation
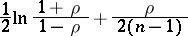 |
and variance  . On this basis one can now define approximate confidence intervals for the true correlation coefficient
. On this basis one can now define approximate confidence intervals for the true correlation coefficient 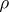 .
.
For the distribution of the sample correlation ratio and for tests of the linearity hypothesis for the regression, see [3].
References
| [1] | H. Cramér, "Mathematical methods of statistics" , Princeton Univ. Press (1946) |
| [2] | B.L. van der Waerden, "Mathematische Statistik" , Springer (1957) |
| [3] | M.G. Kendall, A. Stuart, "The advanced theory of statistics" , 2. Inference and relationship , Griffin (1979) |
| [4] | S.A. Aivazyan, "Statistical research on dependence" , Moscow (1968) (In Russian) |
http://bbs.sciencenet.cn/blog-107667-588530.html
[2] 毛西德格的瓷器密码:神马乖乖?
http://blog.sciencenet.cn/blog-107667-518968.html
http://blog.sciencenet.cn/home.php?mod=space&uid=107667&do=blog&id=657534
http://link.springer.com/article/10.1007%2Fs12209-013-1978-8#
https://blog.sciencenet.cn/blog-107667-603297.html
上一篇:科学网“饭桶帮”成立了!!“饭桶帮”又名“翻腾帮”!
下一篇:【照片】[请问] 这些是什么冰晶?