Zero-point energy, also called quantum vacuum zero-point energy, is the lowest possible energy that a quantum mechanicalphysical system may have; it is the energy of its ground state. All quantum mechanical systems undergo fluctuations even in their ground state and have an associated zero-point energy, a consequence of their wave-like nature. The uncertainty principle requires every physical system to have a zero-point energy greater than the minimum of its classical potential well. This results in motion even at absolute zero. For example, liquid helium does not freeze under atmospheric pressure at any temperature because of its zero-point energy.

The concept of zero-point energy was developed in Germany by Albert Einstein and Otto Stern in 1913, as a corrective term added to a zero-grounded formula developed by Max Planck in 1900.^[1][2] The term zero-point energyoriginates from the German Nullpunktsenergie.^[1][2] An alternative form of the German term is Nullpunktenergie (without the s).

Vacuum energy is the zero-point energy of all the fields in space, which in the Standard Model includes the electromagnetic field, other gauge fields, fermionic fields, and the Higgs field. It is the energy of the vacuum, which in quantum field theory is defined not as empty space but as the ground state of the fields. In cosmology, the vacuum energy is one possible explanation for the cosmological constant.^[3] A related term is zero-point field, which is the lowest energy state of a particular field.^[4]

In 1900, Max Planck derived the formula for the energy of a single energy radiator, e.g., a vibrating atomic unit:^[5]

where 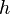is Planck's constant, 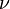is the frequency, k is Boltzmann's constant, and T is the absolute temperature.

Then in 1913, using this formula as a basis, Albert Einstein and Otto Stern published a paper in which they suggested for the first time the existence of a residual energy that all oscillators have at absolute zero. They called this residual energy Nullpunktsenergie (German), later translated as zero-point energy. They carried out an analysis of the specific heat of hydrogen gas at low temperature, and concluded that the data are best represented if the vibrational energy is^[1][2]

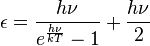
According to this expression, an atomic system at absolute zero retains an energy of ½hν.

Zero-point energy is fundamentally related to the Heisenberg uncertainty principle.^[6] Roughly speaking, the uncertainty principle states that complementary variables (such as a particle's position and momentum, or a field's value and derivative at a point in space) cannot simultaneously be defined precisely by any given quantum state. In particular, there cannot be a state in which the system sits motionless at the bottom of its potential well, for then its position and momentum would both be completely determined to arbitrarily great precision. Therefore, the lowest-energy state (the ground state) of the system must have a distribution in position and momentum that satisfies the uncertainty principle, which implies its energy must be greater than the minimum of the potential well.

Near the bottom of a potential well, the Hamiltonian of a system (the quantum-mechanical operator giving its energy) can be approximated as

where is the minimum of the classical potential well. The uncertainty principle tells us that

making the expectation values of the kinetic and potential terms above satisfy

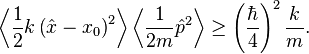
The expectation value of the energy must therefore be at least

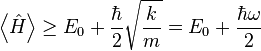
where is the angular frequency at which the system oscillates.

A more thorough treatment, showing that the energy of the ground state actually is requires solving for the ground state of the system. See quantum harmonic oscillator for details.

The concept of zero-point energy occurs in a number of situations.

In ordinary quantum mechanics, the zero-point energy is the energy associated with the ground state of the system. The professional physics literature tends to measure frequency, as denoted by 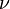above, using angular frequency, denoted with and defined by = . This leads to a convention of writing Planck's constant 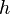with a bar through its top () to denote the quantity 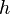/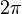. In those terms, the most famous such example of zero-point energy is 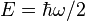associated with the ground state of the quantum harmonic oscillator. In quantum mechanical terms, the zero-point energy is the expectation value of the Hamiltonian of the system in the ground state.

In quantum field theory, the fabric of space is visualized as consisting of fields, with the field at every point in space and time being a quantum harmonic oscillator, with neighboring oscillators interacting. In this case, one has a contribution of 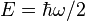from every point in space, resulting in a calculation of infinite zero-point energy in any finite volume; this is one reason renormalization is needed to make sense of quantum field theories. The zero-point energy is again the expectation value of the Hamiltonian; here, however, the phrase vacuum expectation value is more commonly used, and the energy is called the vacuum energy.

In quantum perturbation theory, it is sometimes said that the contribution of one-loop and multi-loop Feynman diagrams to elementary particlepropagators are the contribution of vacuum fluctuations or the zero-point energy to the particle masses.

A phenomenon that is commonly presented as evidence for the existence of zero-point energy in vacuum is the Casimir effect, proposed in 1948 by DutchphysicistHendrik B. G. Casimir (Philips Research), who considered the quantized electromagnetic field between a pair of grounded, neutral metal plates. The vacuum energy contains contributions from all wavelengths, except those excluded by the spacing between plates. As the plates draw together, more wavelengths are excluded and the vacuum energy decreases. The decrease in energy means there must be a force doing work on the plates as they move. This force has been measured and found to be in good agreement with the theory. However, there is still some debate on whether vacuum energy is necessary to explain the Casimir effect. Robert Jaffe of MIT argues that the Casimir force should not be considered evidence for vacuum energy, since it can be derived in QED without reference to vacuum energy by considering charge-current interactions (the radiation-reaction picture).^[7]

The experimentally measured Lamb shift has been argued to be, in part, a zero-point energy effect.^[8]

In cosmology, the zero-point energy offers an intriguing possibility for explaining the speculative positive values of the proposed cosmological constant. ^[9] In brief, if the energy is "really there", then it should exert a gravitational force.^[10] In general relativity, mass and energy are equivalent; both produce a gravitational field. One obvious difficulty with this association is that the zero-point energy of the vacuum is absurdly large. Naively, it is infinite, because it includes the energy of waves with arbitrarily short wavelengths. But since only differences in energy are physically measurable, the infinity can be removed by renormalization. In all practical calculations, this is how the infinity is handled. It is also arguable^{[original research?]} that undiscovered physics relevant at the Planck scale reduces or eliminates the energy of waves shorter than the Planck length, making the total zero-point energy finite.^{[citation needed]}

As a scientific concept, the existence of zero-point energy is not controversial. However, the ability to harness zero point energy for useful work is considered pseudoscience by the scientific community at large.^[11][12]

Over the years, there have been numerous claims of devices capable of extracting usable zero-point energy. None of the claims have ever been confirmed by the scientific community, and most of these claims are dismissed by physicists and engineers after third-party inspection of such a device or based on disbelief in the viability of a technical design and theoretical corroboration.^[13]

Science skeptic and writer, Martin Gardner has called claims of such zero-point-energy-based systems, "as hopeless as past efforts to build perpetual motion machines"^[14]Perpetual motion machine refers to technical designs of machines that can operate indefinitely, optionally with additional output of excessive energy, without any cited input source of energy, which is in violation of the laws of thermodynamics. Formally, technical designs that claim to harness zero-point energy would not fall into this category, while zero-point energy is claimed as the input source of energy. However, in popular science it is common^{[weasel words]} to disregard whether such a technical claim deviates first and foremost from the laws of thermodynamics, or from the theories of quantum mechanics. Despite the scientific stance to typically discount the claims, numerous articles and books have been published addressing and discussing the potential of tapping zero-point-energy from the quantum vacuum or elsewhere. Examples of such are the work of the following authors: Claus Wilhelm Turtur,^[15] Jeane Manning, Joel Garbon,^[16] John Bedini,^[17] Tom Bearden,^[18][19][20] Thomas Valone,^[21][22][23] Moray B King,^[24][25][26] Christopher Toussaint, Bill Jenkins,^[27]Nick Cook^[28] and William James.^[29]

In quantum theory, zero-point energy is a minimum energy below which a thermodynamic system can never go.^[11] Thus, none of this energy can be withdrawn without altering the system to a different form in which the system has a lower zero-point energy. One of the hypotheses that claims that zero-point energy is infinite is stochastic electrodynamics. In it, the zero-point field is viewed as simply a classical background isotropic noise wave field which excites all systems present in the vacuum and thus is responsible for their minimum-energy or "ground" states. The requirement of Lorentz invariance at a statistical level then implies that the energy density spectrum must increase with the third power of frequency, implying infinite energy density when integrated over all frequencies.^[30]

According to a NASA contractor report, "the concept of accessing a significant amount of useful energy from the ZPE gained much credibility when a major article on this topic was published in Aviation Week & Space Technology (March 1st, 2004), a leading aerospace industry magazine".^[31]

The calculation that underlies the Casimir experiment, a calculation based on the formula predicting infinite vacuum energy, shows the zero-point energy of a system consisting of a vacuum between two plates will decrease at a finite rate as the two plates are drawn together. The vacuum energies are predicted to be infinite, but the changes are predicted to be finite. Casimir combined the projected rate of change in zero-point energy with the principle of conservation of energy to predict a force on the plates. The predicted force, which is very small and was experimentally measured to be within 5% of its predicted value, is finite.^[32] Even though the zero-point energy is theoretically infinite, there is as yet no evidence to suggest that infinite amounts of zero-point energy are available for use, that zero-point energy can be withdrawn for free, or that zero-point energy can be used in violation of conservation of energy.^[33]

In the contrary of energy generation, a field of study where there is a somewhat realistic potential for the utilization of zero-point energy might be in the design of extremely small scale devices like MEMS and NEMS or in distant futuristic propulsion technology of extremely long-distance space-travel.^[11]

A document released by the NGIC shows there is ongoing worldwide research into zero-point energy, particular in China, Germany, Russia and Brazil. An analyst of the Defense Intelligence Agency has indicated that research into successfully harnessing zero-point energy for energy generation purposes is a serious concern inside the intelligence community.^[11]

In 1986, Arthur C. Clarke published a science fiction novel called The Songs of Distant Earth which depicts a starship called Magellan that is powered by the quantum vacuum zero point energy.

In Disney/Pixar's animated film The Incredibles, the main villain Syndrome refers to his weapons as using zero-point energy.^[34][35] The fan fiction community devoted to the character is named "Zero Point" because of this.^[36]

In the critically acclaimed game from Valve Corporation, Half-Life 2, a "zero-point energy field manipulator" (popularly known as a "gravity gun"), meant to handle sensitive, anomalous and hazardous materials, is used as both a weapon to throw objects at enemies in high speeds, as a primary attack, and a tool to solve physics puzzles consisting in moving objects of considerable weight.

In Nintendo's Star Fox series, there are mentions of a "G-Diffusion" system. The so-called G-diffusion is an experimental power system used in the game's starfighters that reduces gravity forces on the pilot and provides a respectable power source for shields and propulsion, using advanced zero-point energy technology.

In the webcomic Schlock Mercenary, a major running plot-point centers around an enormous zero-point energy generator built from the super massive black hole comprising the core Milky Way Galaxy.

In the popular science-fiction programs generally labeled under Stargate SG-1, the intergalactic colonists visiting Atlantis often refer to and make use of "ZPMs" shortened from "Zero-Point Modules." Although fantastically powerful in terms of available energy density, these fictional energy sources still dissipate over time which the example of Helium's non-solid state proves inaccurate.

理论上，QHA比HA多考虑了一个体积效应。