博文

自由电子费米能级之问答

已有 5329 次阅读 2013-11-23 00:06 |个人分类:电子结构计算|系统分类:科研笔记

关注：

1）怎样理解 the DOS for equivaalent free-electron systems.

2) 怎样清楚明了的问答问题。

答：

Dear Xiao-qiu,

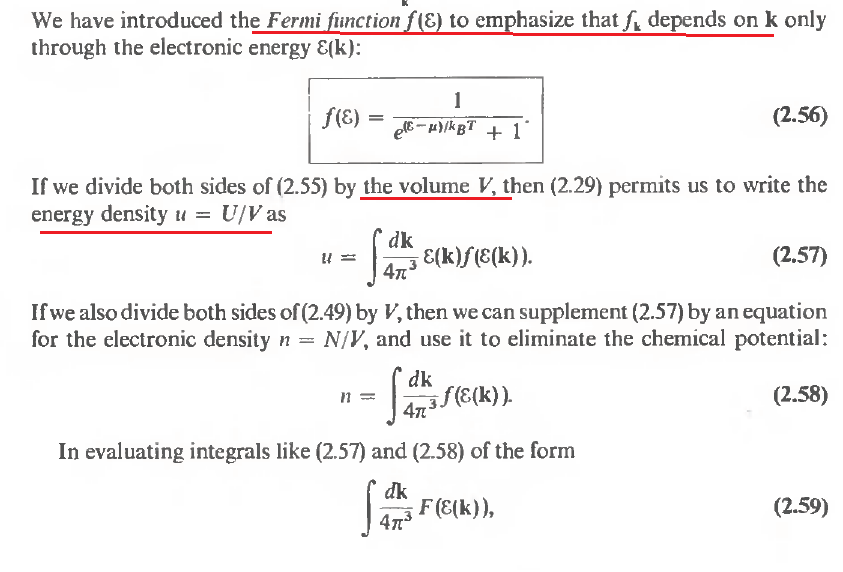
The formulas you have there are correct, but you also need the equation for the general density of states of the free 3D electron gas. That is found, for example, in Neil’s book, chapter 2 (equation 2.63 in my German edition):

DOS(E) = 3/2*N/Ef*sqrt(E/Ef).

The way to obtain a plot such as in my figures below is as follows:

1. Set Ef to the bandwidth of the actual DOS (around 60eV in my case below).【为什么】

2. Set N=1. （表明对单电子情形，处理的晶胞中有几个电子除以几）

3. Plot DOS(E) as written above, but with an energy offset of -Ef.

【DOS(E) = -3/2*N/Ef*sqrt(-（E-Ef)/Ef).】 Ef取费米能级以下的带宽，取负值。

To judge a “good” metal is not so easy, even the DOS only gives you indirect information. In my opinion, a “good” metal is a good electric conductor, so technically you would have to calculate the conductivity. That is beyond our scope usually, so we say that if the DOS is “high” at the Fermi energy (maybe 0.3-0.5 or higher with respect to the free electron value) than we assume that the conductivity will also be high.

Hope that helps, let me know if there are any issues.

Best wishes,

Andreas

问：

Dear Andreas,

First of all, I hope everything goes very well with you.

I met a small problem when I read your paper recently, as shown in the picture below. Could you briefly tell me:

1) How to get the free-electron DOS per electron with a certain bandwidth? For example, I also want to plot the free-electron DOS and the DOS　of scandium hydrides together to check whether the hydrides are good or poor metals. I got these two equations from the textbook, but I don’t know how to use them to solve this problem exactly.