求教心切。以下内容，词不达意。敬请指教！敬请批评！

同伦（Homotopy）：统一场的一个下流解！

孙冰老师：您把俺吓尿了！

     真傻昨天的博文《反思麦克斯韦经典电磁理论宣言》贴出之后，真的达到了“求教”目的！

孙冰老师

http://blog.sciencenet.cn/home.php?mod=space&uid=3388899

2018-8-30 08:04 写到：

今天俺看到时，顿时惊呆了！三观尽毁！！

什么是物理学家要的“统一”？

什么是“统一”？

wiki 百科里给出的圆锥曲线的一般形式：

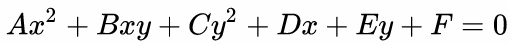
矩阵形式：

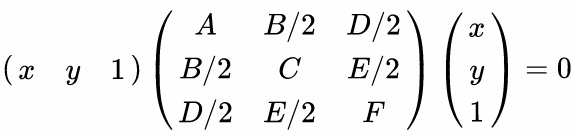
可是，常见二次曲线的标准形式为：

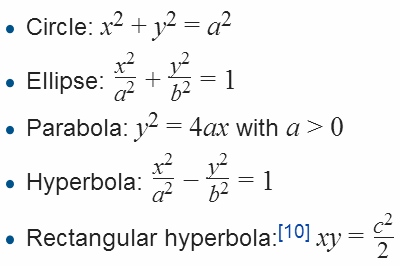
困惑：

什么是“统一”？

此外，还有胡新平老师2016年的统一《一、二次曲线的轨迹统一及性质》。

同伦（Homotopy）：统一场的一个下流解！

https://en.wikipedia.org/wiki/Homotopy

百度百科对“函数的同伦”的解释：

苏联《数学百科全书》扩展版(wiki版)里说：

https://www.encyclopediaofmath.org/index.php/Homotopy

      A formalization of the intuitive idea of deformability of one mapping into another. More exactly, two mappings f and g are called homotopic (denoted by f ~ g ) if there exists a family of continuous mappings ……

    把电磁理论作为一端，把引力理论作为另一端，用一个同伦（Homotopy）之后，引力-电磁居然被这样下流地统一了！

    二次曲线，或多或少就是被这样“下流”地写出了一般的形式！！

统一：小心毁三观！

    奥卡姆剃刀（简约法则，Occam's razor，Ockham's razor，Ocham's razor，law of parsimony），到底该怎么理解？

    简洁与美，美与真， ……，诸多观念，到底该怎么理解？

    像二元二次曲线的一般形式一样，统一引力和电磁，算是统一吗？什么是我们想要的统一？

    【用“泄露”的电磁相互作用，来表示“引力相互作用”：类似气体分子的瞬时偶极（instantaneous dipole）。】是真傻几年前给出的“物理”统一。

    【或许在别的拟合函数形式下，引力-电磁的统一会变得容易？】是真傻2018-01-15之前给出的“数学”统一。

最后，真诚地感谢并祝福《科学网》！祝《科学网》越办越好！

《科学网》，就是我们的“奥林匹亚科学院（Akademie Olympia，Olympia Academy）”！

比 Conrad Habicht，Maurice Solovine 和 Albert Einstein 更好的奥林匹亚科学院！

相关链接：

[1] 圆锥曲线，百度百科

https://baike.baidu.com/item/%E5%9C%86%E9%94%A5%E6%9B%B2%E7%BA%BF

[2] Conic section, From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Conic_section

[3] 同伦，百度百科

https://baike.baidu.com/item/%E5%90%8C%E4%BC%A6

[4] Homotopy. Encyclopedia of Mathematics

https://www.encyclopediaofmath.org/index.php/Homotopy

[5] Homotopy, mathematics, Britannica.com

https://www.britannica.com/science/homotopy

[6] Homotopy, From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Homotopy

[7] Homotopy, from Wolfram MathWorld

http://mathworld.wolfram.com/Homotopy.html

[8] 胡新平. 一、二次曲线的轨迹统一及性质[J]. 数学通报, 2016, 55(12): 47-51, 54.

[9] 奥卡姆剃刀原理，百度百科

https://baike.baidu.com/item/%E5%A5%A5%E5%8D%A1%E5%A7%86%E5%89%83%E5%88%80%E5%8E%9F%E7%90%86

[10] Occam's razor, From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Occam%27s_razor

[11] Occam's razor, philosophy, Britannica.com

https://www.britannica.com/topic/Occams-razor

[12] 2018-08-29，反思麦克斯韦经典电磁理论宣言

http://blog.sciencenet.cn/blog-107667-1131694.html

[13] 2018-01-15（2012-04-12），SI基本单位中安培定义的两种可能缺陷----中科院科学智慧火花

http://idea.cas.cn/viewdoc.action?docid=4681

[14] Olympia Academy, From Wikipedia, the free encyclopedia

https://en.wikipedia.org/wiki/Olympia_Academy

感谢您的指教！

感谢您指正以上任何错误！

感谢您提供更多的相关资料！