[重大困惑] 为什么正态分布随机数不能“被”预测

下面是直观的叙述。不知道怎样专业地描述。

一、重大困惑

   在“时间序列分析与预测”里，通常都把“正态分布”作为“不可预测”量。

   这是为什么？

   这里的“预测”，是指“瞬时值”的预测；不是“统计量”的预测。平稳时间序列的“统计量”不随时间变化。

   难道只有“独立同分布的正态分布”时间序列里没有了“信号”？

   难道其它的“独立同分布的随机变量”里还有可以预测的“信号”？

   实在想不明白。一时也没有找到权威的解释。

二、请教

   （1）正态分布时间序列的延迟自相关系数（lag >1），都是0吗？

   均匀分布时间序列的延迟自相关系数（lag >1），也都是0吗？

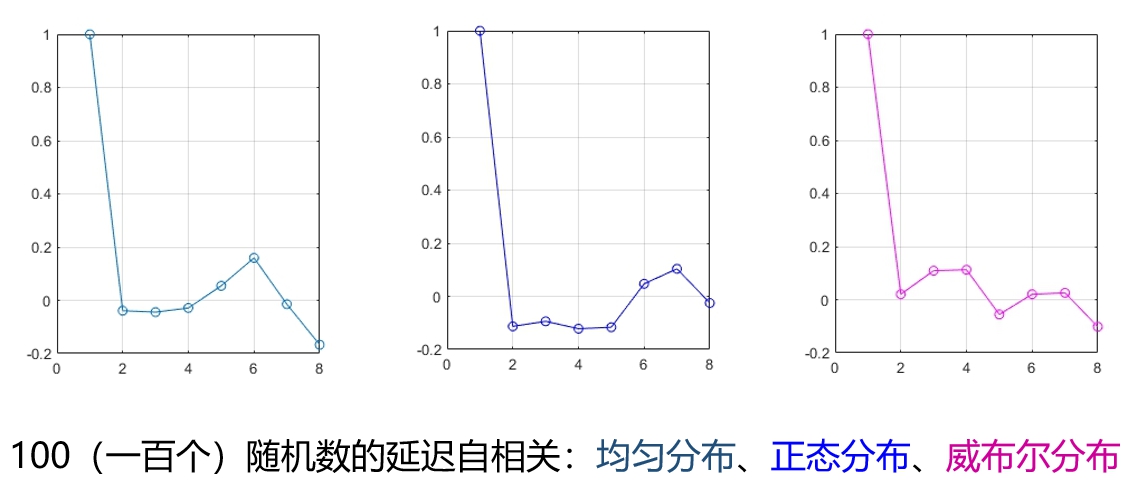
上面2图是延迟自相关系数的一些数字的仿真结果

   （2）其它分布的随机数，可以分解成若干个“独立同分布的正态分布随机数”之和吗？

   好像有类似的说法。

三、正态分布是“最基础”的随机数？

   就是不能用别的随机数表示出？

参考资料：

[1] 范剑青，姚琦伟. 非线性时间序列：建模、预报及应用[M]. 北京：高等教育出版社，2005.

[1-2] Jianqing Fan, Qiwei Yao. Nonlinear Time Series: Nonparametric and Parametric Methods (Springer Series in Statistics)[M]. 1st. Springer, 2003.

[3] Harald Cramér, 1961, On some classes of Non-Stationary Processes. Berkeley Symposium on Mathematical Statistics and Probability, University of California Press, Vol. 4.2, 57-78 (1961). Fourth Berkeley Symposium on Mathematical Statistics and Probability

June 20-July 30, 1960. Statistical Laboratory of the University of California, Berkeley

https://projecteuclid.org/ebooks/bsmsp/Proceedings-of-the-Fourth-Berkeley-Symposium-on-Mathematical-Statistics-and/toc/bsmsp/1200512587

[4] Libres pensées d'un mathématicien ordinaireLPMO - Djalil Chafaï, 2021-04-28, Tutorial on large deviation principles

https://djalil.chafai.net/blog/2018/03/

[5] Carl Harald Cramér, MacTutor History of Mathematics Archive

https://mathshistory.st-andrews.ac.uk/Biographies/Cramer_Harald/

[6] Charles Annis, 2014-06-08, Sums of Random Variables (uniform distribution)

http://www.statisticalengineering.com/sums_of_random_variables.htm

[7] Charles Annis, 2014-06-08, Distributional Inter-Relationships

http://www.statisticalengineering.com/distributions.htm

[8] Uniform distribution. Encyclopedia of Mathematics.

https://encyclopediaofmath.org/wiki/Uniform_distribution

相关链接：

[1] 2021-07-19，[资料] 时间序列分析与预测的常用误差统计指标

http://blog.sciencenet.cn/blog-107667-1296109.html

[2] 2021-06-15，[求助] 时间序列预测里的回归模型“定阶”、“样本容量”的优化方法

http://blog.sciencenet.cn/blog-107667-1291316.html

[3] 2021-02-05，[笔记] 时间序列预测里的一些“专业术语”

http://blog.sciencenet.cn/blog-107667-1270762.html

[4] 2019-06-08，[学习资料搜集] 时间序列（time series）学习书籍

http://blog.sciencenet.cn/blog-107667-1183775.html

[5] 2016-03-07，关于时间序列的“组合预测之谜 forecast combination puzzle”

http://blog.sciencenet.cn/blog-107667-961080.html

[6] 2012-05-28，综合预测、组合预测

http://blog.sciencenet.cn/blog-107667-575951.html

[7] 2010-11-07，复杂系统行为预测的“机理+辨识”策略

http://blog.sciencenet.cn/blog-107667-381404.html

[7-2] 杨正瓴，张军，陈曦，等. 复杂系统行为预测的“机理+辨识”策略[EB/OL]. 北京：中国科技论文在线 [2006-09-29]. http://www.paper.edu.cn/releasepaper/content/200609-432

[8] 2018-08-18，“大数据”时期，更渴望“小样本数理统计学”

http://blog.sciencenet.cn/blog-107667-1129894.html

[9] 2019-12-03，[求证] 噪声有益成因机理分析的国际优先权

http://blog.sciencenet.cn/blog-107667-1208653.html

[10] 2020-03-26，现实中常见的概率分布

http://blog.sciencenet.cn/blog-107667-1225390.html

[11] 2019-07-27，威布尔分布 Weibull Distribution 资源网页搜集

http://blog.sciencenet.cn/blog-107667-1191323.html

[12] 2019-09-27，极值分布 Extreme Values Distribution 相关网页

http://blog.sciencenet.cn/blog-107667-1199726.html

感谢您的指教！

感谢您指正以上任何错误！

感谢您提供更多的相关资料！