【开篇语】

微分概念在整个微积分体系中占有重要地位。理解微分概念是微积分教育的重要环节。在历史上，微分的定义经历了很长时间的发展。牛顿、莱布尼兹是微积分的主要创建人，他们的微积分可以称为第一代微积分，第一代微积分的方法是没有问题的，而且获得了巨大的成功，但是对微分的定义（即微分的本质到底是什么）的说明不够清楚；以柯西、维尔斯特拉斯等为代表的数学家在极限理论的基础上建立了微积分原理，可以称之为第二代微积分，并构成当前教学中微积分教材的主要内容。第二代微积分与第一代微积分在具体计算方法上基本相同，第二代微积分表面上解决了微分定义的说明，但是概念和推理繁琐迂回。

当前，围绕微分定义问题，国内外学术界已经开始形成一些讨论，参与者从科学院院士，中青年数学工作者，以致在读博士硕士，当然也包括一些毫无话语权的“N无数学家”。但真理面前人人平等，只要我们抱着持之有故言之成理的科学态度，相信会引发深刻的思考。

为了使得微分定义的讨论更加深入，并且有充足的养料支撑，有必要将古今中外现行微积分学术著作中的微分定义详细调查。从今天起，我将在我所搜集整理的微积分定义逐次摘录在网上，方便大家讨论。在摘录的同时，将做一些简单的讨论。

【法国】

First let us recall what a derivative is. Consider a function $f$ with complex values defined on an interval $\mathrm{I} \subset \mathbb{R}$ not reducing to a single point. Given an$\mathrm{a} \in I$, we examine the behaviour of $\mathrm{f}(\mathrm{a}+\mathrm{h})$ as $\mathrm{h}$ tends to 0, implicitly assuming, in all that follows, that $\mathrm{a}+\mathrm{h}$ remains in $\mathrm{I}$. If $\underline{f}$ is continuous at the point $\mathrm{a}$, then $\mathrm{f}(\mathrm{a}+\mathrm{h})$ tends to $\mathrm{f}(\mathrm{a})$, in other words, the function $\mathrm{f}$ is “almost constant” on a neighbourhood of $\mathrm{a}$ as we explained profusely in $\mathrm{n}^{0} 2$.

But instead of approximating $f$ on a neighbourhood of $a$ by the constant function $x \mapsto f(a)$, one might try to approximate it by a function a little less simple, for example a linear function of the form $g(x)=c x+d$.

The least that one can ask is that it should be equal to $f$ at the point $a$, whence the condition $c a+d=f(a)$. Then

(14.1) $g(x)=c(x-a)+f(a)$,

whence

(14.2) $g(a+h)=c h+f(a)$.

It remains to choose the constant $C$ as well as possible.

Now the error committed in replacing f by $g$ is given by

$f(a+h)-g(a+h)=f(a+h)-f(a)-c h=h\left[\frac{f(a+h)-f(a)}{h}-c\right]$.

To minimise this we should choose $c$ so that the coefficient of $h$ is as small as possible and, even better, tends to 0 with $h$. This means that we must choose

(14.3) $c=\lim _{h \rightarrow 0 \atop h \neq 0} \frac{f(a+h)-f(a)}{h}$.

When this limit exists-and, of course, it does not always do so-, we say that $c$ is the derivative of f at $a$, denoted by $f^{\prime}(a)$; so the best way of approximating $f$ on a neighbourhood of $a$ by a linear function is to choose the function

(14.4) $y=f^{\prime}(a)(x-a)+f(a)$;

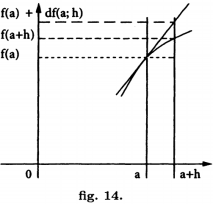
this is the linear tangent function to f at $a$, so called because (4) is the equation of the tangent line to the graph of f at the point $(a, f(a))$ of the plane. Its homogeneous part in $h=x-a$ is called the differential of $f$ at $a$; it depends both on the point $a \in I$ and on an auxiliary variable $h \in \mathbb{R}$, whence the notation $a \in I$ to denote the function $h \mapsto f^{\prime}(a) h$ and the notation

(14.5) $d f(a ; h)=f^{\prime}(a) h$

to denote its value at $a$. This mode of presenting derivatives and differentials is already essentially to be found in Weierstrass.

If for example $f(x)=x$, then $f^{\prime}(a)=1$, so that the differential of $f$ is the function $h \mapsto h$; in other words

(14.6) $d x(a ; h)=h$

for all $a$ and $h$ real. Comparing with (5), we see that

(14.7) $d f(a ; h)=f^{\prime}(a) d x(a ; h)$

for every function $f$ that is dierentiable at$a$; in a more condensed way:

(14.8) $d f(a)=f^{\prime}(a) d x(a)$,

the product of the linear function $d x(a)$, i.e. $h \mapsto h$, by the constant $f^{\prime}(a)$ (relative to $h$); and since in fact the differential $d x(a)$ does not depend on $a$, one may as well call it $dx$ for short, and obtain the formula

(14.9) $d f(a)=f^{\prime}(a) d x$,

or even, if $f$ is differentiable at any $x \in I$,

(14.10) $d f(x)=f^{\prime}(x) d x$ or simply $d f=f^{\prime}(x) d x$.

Hence the traditional formulation

(14.11) $f^{\prime}(x)=d f / d x$

for derivatives; it has no meaning in this framework since $d f$ and $dx$ are functions and not numbers, but everyone uses it, not only to follow in the tradition, but also, and above all, because of its convenience, particularly at the elementary level.

参考文献：

[1] Roger Godement. Analysis I: Convergence, Elementary functions[M]. Springer-Verlag Berlin Heidelberg，2004：244-246.